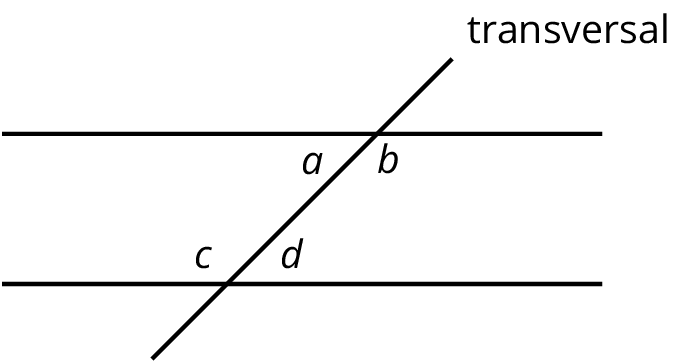
If two interior angles lie on opposite sides of the transversal they are called alternate interior angles.
In the figure, a and d are alternate interior angles, and b and c are also alternate interior angles.
The Pythagorean Theorem is a name for the property of all right triangles that the square of the hypotenuse is equal to the sum of the squares of the legs. It is often expressed as a^2+b^2=c^2.
Interior angles are angles that are made by a transversal crossing two parallel lines. They are the angles that lie between the parallel lines, not outside them.
If two interior angles lie on opposite sides of the transversal they are called alternate interior angles.
In the figure, a and d are alternate interior angles, and b and c are also alternate interior angles.
See dilation.
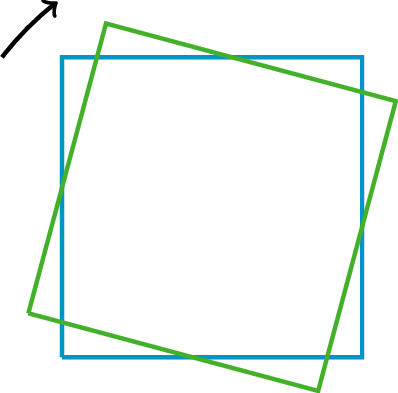
An object is rotating clockwise if it is turning in the same way that the hour or minute hand goes around a clock.
The tilted square is rotated 15^\circ clockwise from the square sitting horizontally on its base.
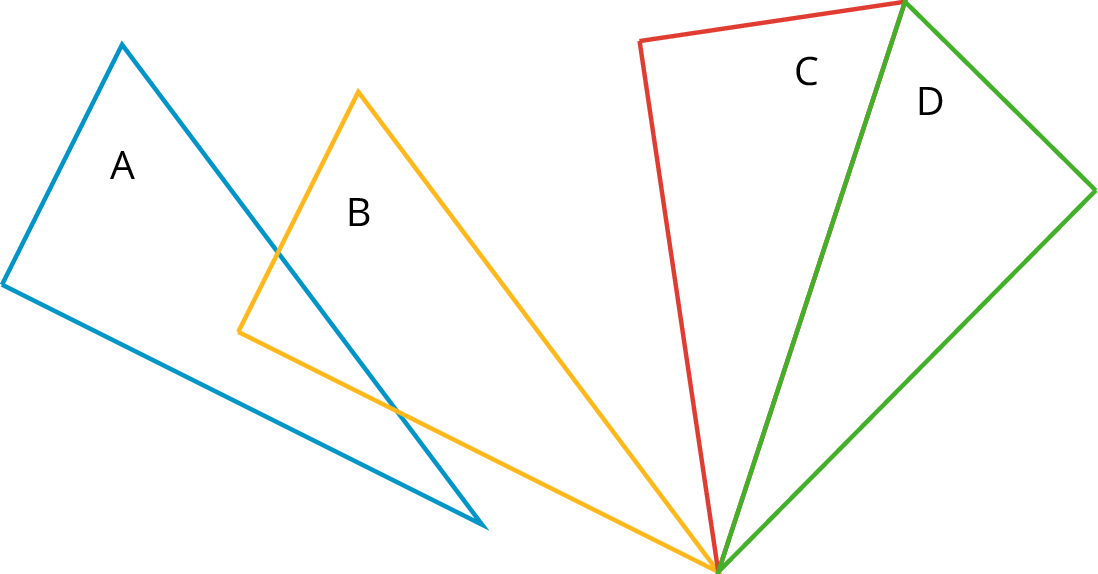
One figure is congruent to another if there is a rigid transformation (a sequence of translations, rotations, and reflections) that moves the first figure so that it fits exactly over the second. The second figure is called the image of the rigid transformation.
Triangle A is congruent to triangle D. A translation takes triangle A to triangle B, a rotation takes triangle B to triangle C, and a reflection takes triangle C to triangle D.
In an expression like 5x + 2 the number 2 is called the constant term because it doesn't change when x changes.
If a part of the original figure matches up with a part of the copy, we call them corresponding parts. The part could be an angle, point, or side, and you can have corresponding angles, corresponding points, or corresponding sides.
If you have a distance between two points in the original figure, then the distance between the corresponding points in the copy is called the corresponding distance.
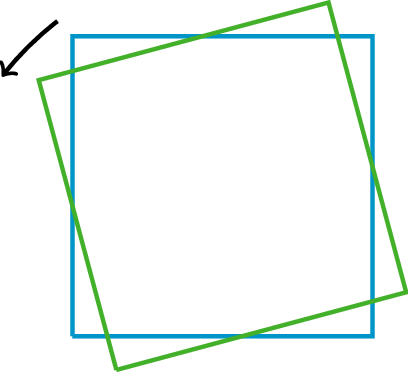
An object is rotating counterclockwise if it is turning in the opposite way to the way that the hour or minute hand goes around a clock.
The tilted square is rotated 15^\circ counterclockwise from the square with a horizontal base.
The cube root of a number x, written \sqrt[3]{x}, is the number whose cube is x. For example, \sqrt[3]{8} = 2 because 2^3 = 8. The cube root of 0 is 0.
A variable representing the output of a function.
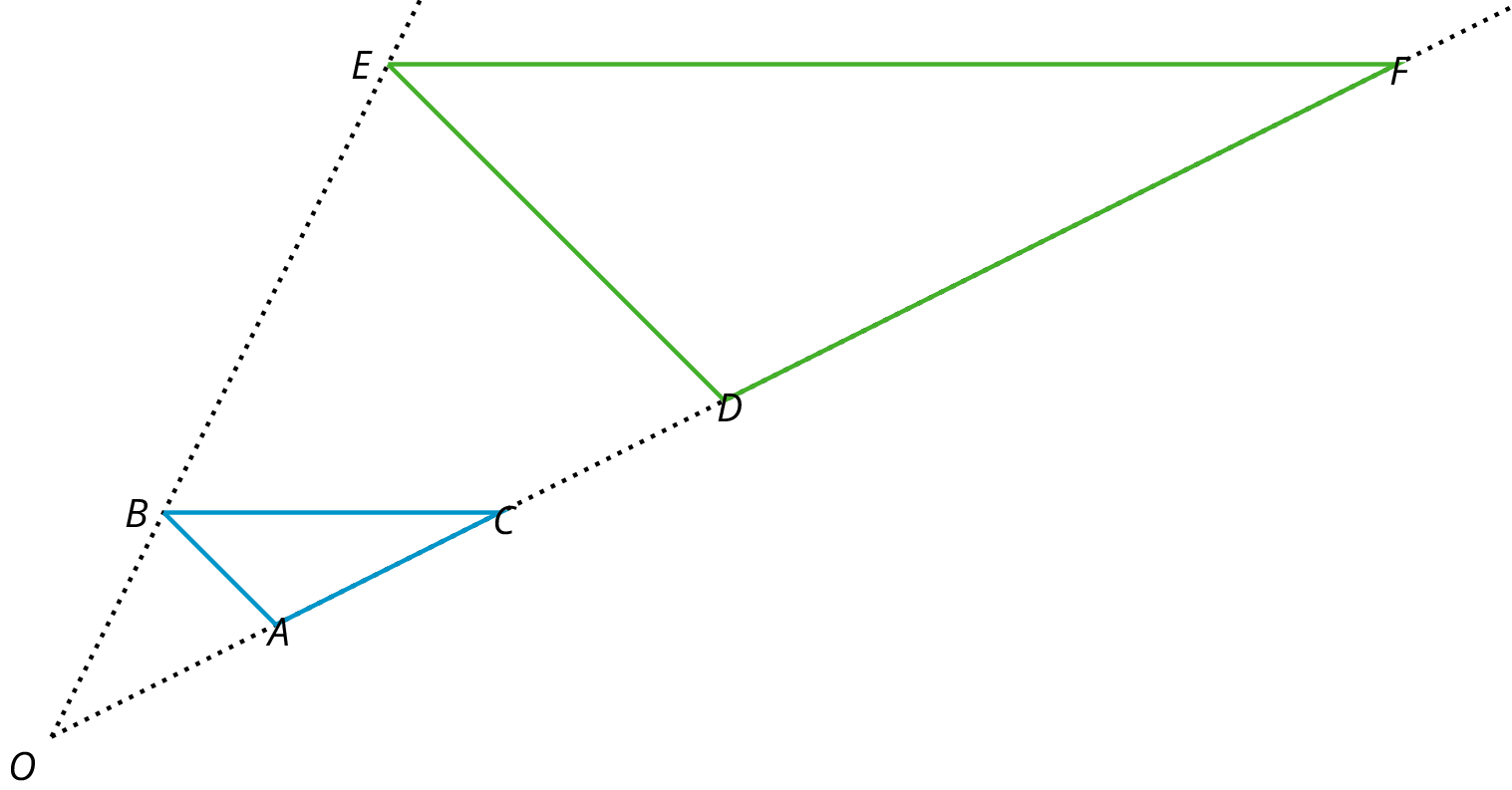
A dilation with center O and positive scale factor r takes a point P along the line OP to another point whose distance is r times further away from O than P is. If r < 1 then the new point is really closer to O, not further away.
The triangle DEF is a dilation of the triangle ABC with center O and with scale factor 3. So D is 3 times further away from O than A is, E is 3 times further away from O than B is, and F is 3 times further away from O than C is.
A function is a rule that assigns to each allowable input exactly one output.
In a right triangle, the side opposite the right angle is called the hypotenuse.
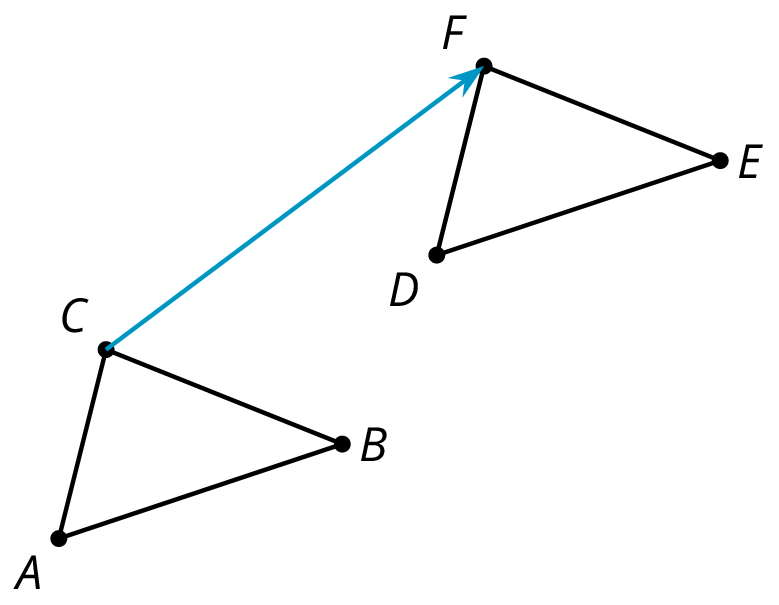
Translations, rotations, and reflections move objects in the plane. Points, segments, and other parts of the original all have corresponding parts on the “moved object.” The moved object is called the image.
For example, here is triangle ABC and a translation to the right and up which is labeled DEF.
Point F in the image corresponds to point C, segment EF in the image corresponds to segment BD, and angle DEF corresponds to angle ABC.
A variable representing the input of a function.
An irrational number is a number that is not rational. That is, it cannot be expressed as a positive or negative fraction, or zero.
In a right triangle, the sides that are adjacent to the right angle are called the legs.
A linear relationship is a relationship between two quantities where one quantity has a constant rate of change with respect to the other. The relationship is called linear because its graph is a line. A linear relationship can be represented by an equation of the form y = mx + b, where m and b are constants.
See association.
In statistics, an outlier is a data value that is way outside the group of other values.
See association.
In a linear relationship between two quantities x and y, with equation y = mx + b, the constant m is the rate of change. It tells you how much y changes when x changes by 1. It is also the slope of the graph of the relationship.
A rational number is a fraction or the opposite of a fraction. Remember that a fraction is a point on the number line that you get by dividing the unit interval into b equal parts and finding the point that is a of them from 0. We can always write a fraction in the form \frac{a}{b} where a and b are whole numbers, with b not equal to 0, but there are other ways to write them. For example, 0.7 is a fraction because it is the point on the number line you get by dividing the unit interval into 10 equal parts and finding the point that is 7 of those parts away from 0. We can also write this number as \frac{7}{10}.
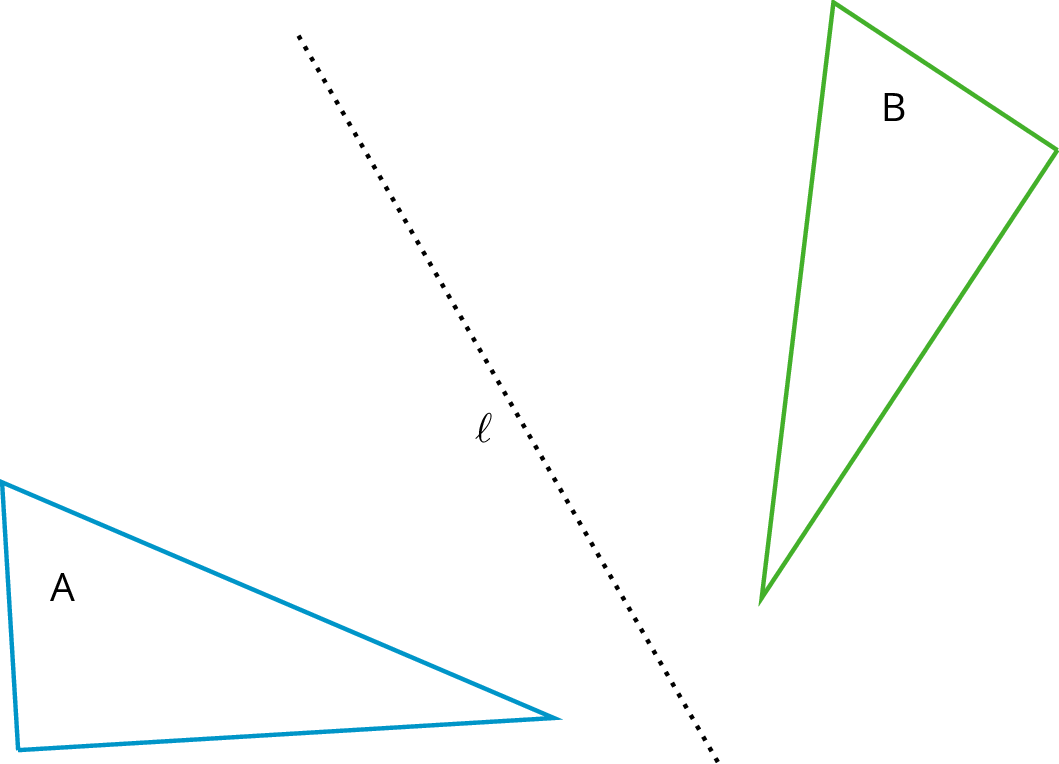
The reflection of a figure across a line takes every point of the figure to a point directly opposite to it on the other side of the line and the same distance from the line. In the figure, the triangle B is the reflection of the triangle A across the line \ell.
See frequency.
A rigid transformation is a sequence of translations, rotations, and reflections. If a rigid transformation is applied to a geometric figure, the resulting figure is called the image of the original figure under the transformation.
The diagram shows a rigid transformation consisting of a translation (from A to B) followed by a rotation (from B to C) followed by a reflection (from C to D). The last triangle is the image of the first triangle under this rigid transformation.
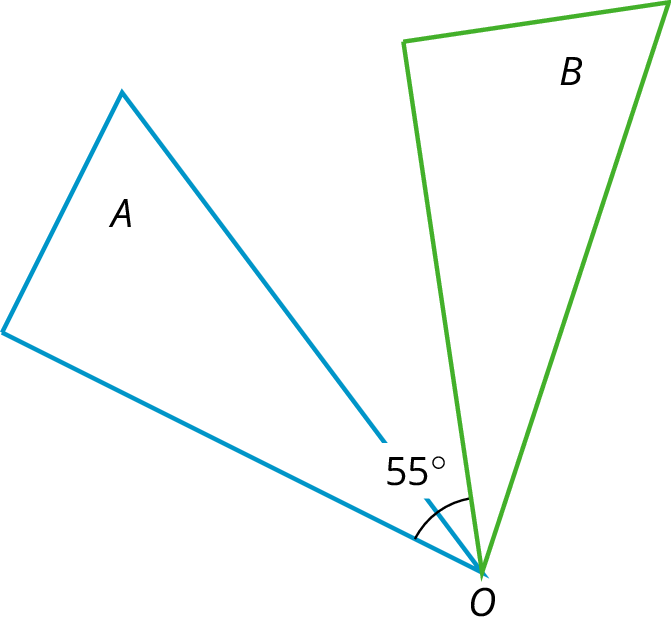
A rotation has a center, an angle, and a direction. It moves every point of a figure in a circle around the center, in the direction specified (clockwise or counterclockwise), and for a distance specified by the angle. For example, in the figure, triangle A is rotated 55^\circ clockwise about center O to get triangle B.
A scatter plot shows data points for the relationship between two variables on a pair of coordinate axes, one for each variable.
Scientific notation is a way of writing very large or very small quantities using powers of 10. A number in scientific notation is the product of two factors:
It is standard for numbers in scientific notation to use the \times symbol to indicate multiplication between these two factors.
A segmented bar graph compares two categorical variables by showing one of the categories on the horizontal axis and representing the percentages in the other category with stacked vertical bars.
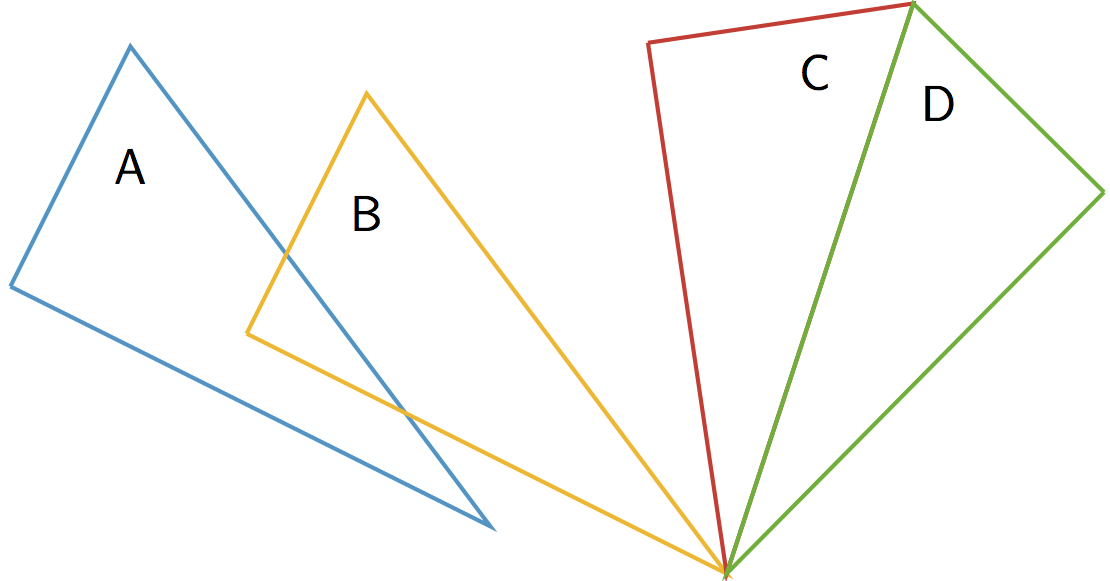
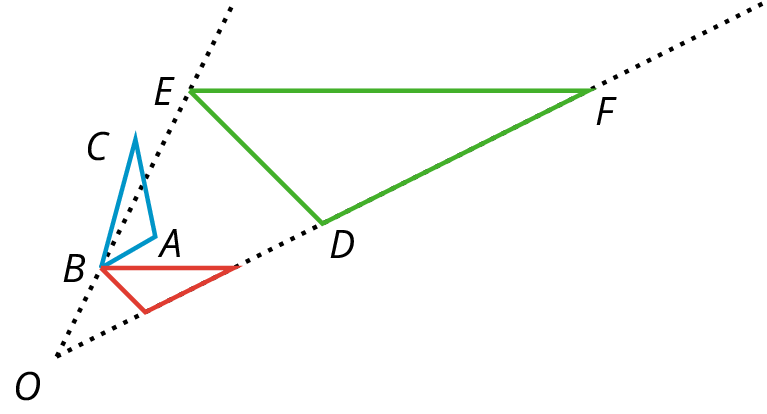
A sequence of transformations is a set of translations, rotations, reflections, and dilations performed in a particular order on a geometric figure, resulting in a final figure.
The diagram shows a sequence of transformations consisting of a translation (from A to B) followed by a rotation (from B to C) followed by a reflection (from C to D). The last triangle is the final figure resulting from the sequence.

One figure is similar to another if there is a sequence of rigid transformations and dilations that moves the first figure so that it fits exactly over the second.
Triangle ABC is similar to triangle DEF because a rotation about B followed by a dilation with center O takes the first triangle to the second.
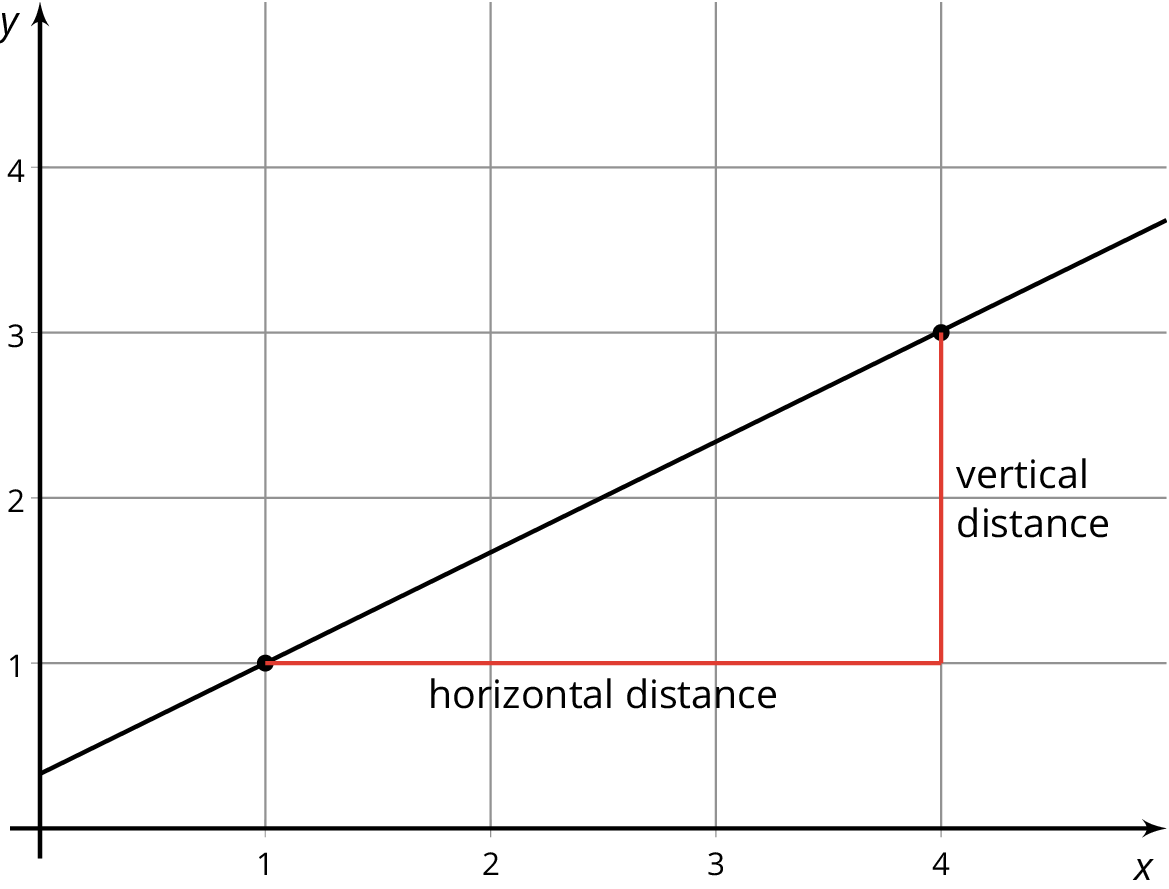
The slope of a line is the quotient of the vertical distance and the horizontal distance between any two points on the line.
A solution to an equation with two variables is any pair (x,y) that can be used in place of the variables to make the equation true.
The square root of a positive number x, written \sqrt{x}, is the positive number whose square is x. For example, \sqrt{4} = 2 because 2^2 = 4 and 2 is positive. The square root of 0 is 0.
If the two rays that make an angle form a straight line, we call the angle a straight angle.
A system of equations is a set of equations where you want to find a solution that makes all the equations true at the same time. In these materials it is a pair of two linear equations in two variables.
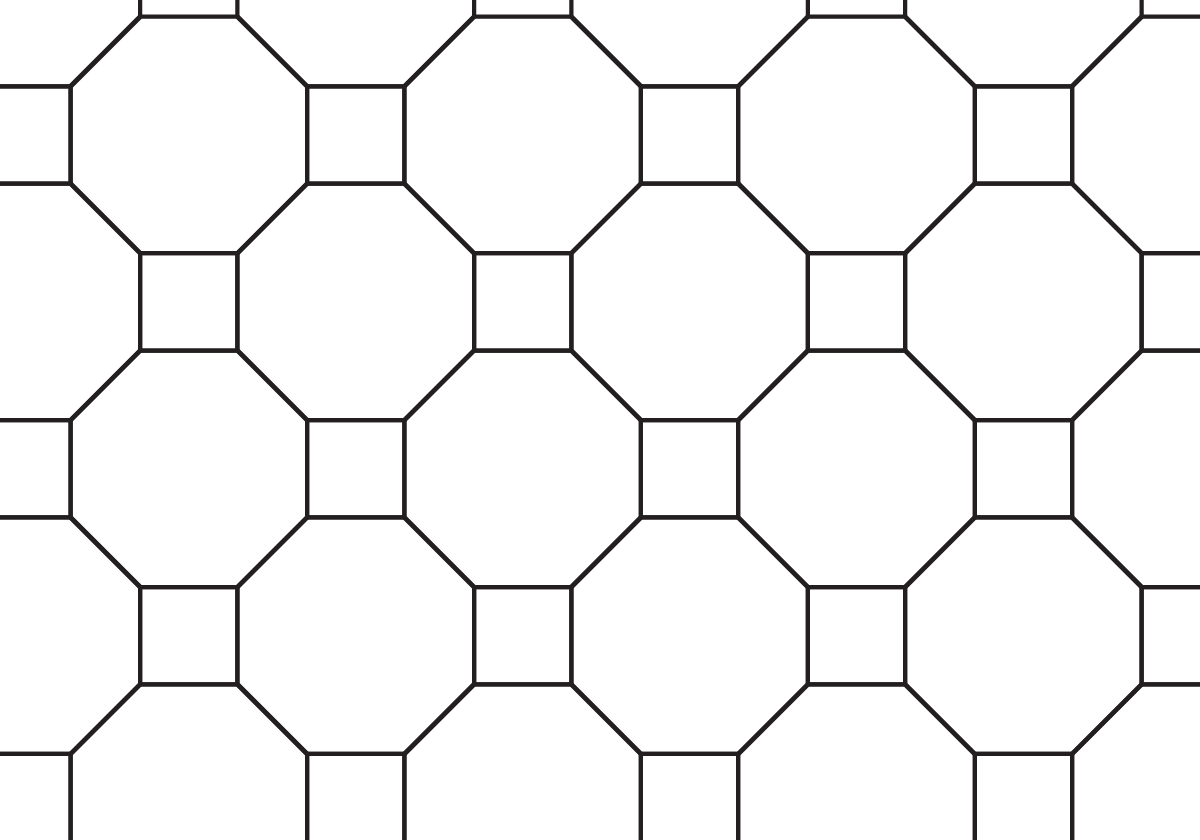
A tessellation of the plane is a regular repeating pattern of one or more shapes that covers the entire plane.
A transformation is a translation, rotation, reflection, or dilation, or combination of these. There is also a more general concept of a transformation of the plane that is not discussed in grade 8.
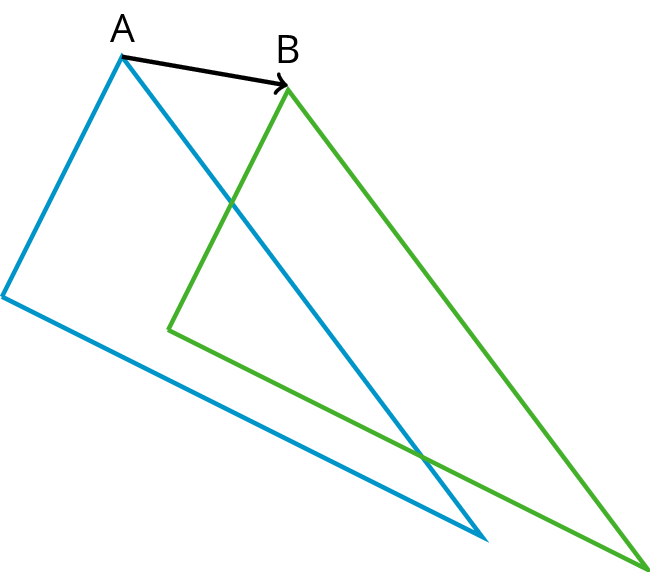
A translation has a distance and a direction. It moves every point in a figure the given distance in the given direction.
The figure on the left is translated to the figure on the right in the direction from A to B, using the distance from A to B.
A transversal to two parallel lines is a line that cuts across them, intersecting each one.
A two-way table provides a way to investigate the connection between two categorical variables.
It shows one of the variables across the top and the other down the side. Each entry in the table is the frequency or relative frequency for the corresponding pair of categories.
The two-way table shows the results of a study of the connection between meditation and state of mind of athletes before a track meet.
| meditated | did not meditate | total | |
|---|---|---|---|
| calm | 45 | 8 | 53 |
| agitated | 23 | 21 | 44 |
| total | 68 | 29 | 97 |
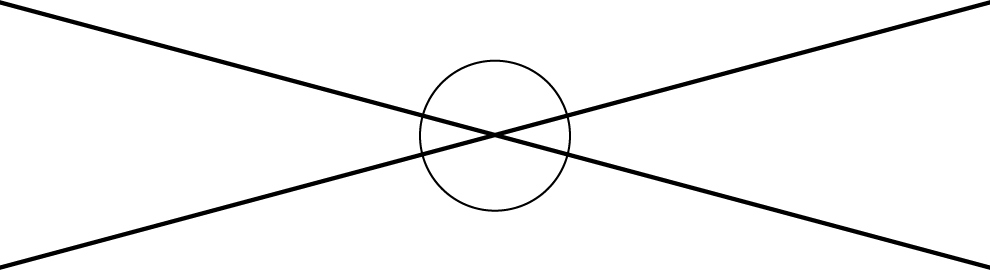
A pair of vertical angles is a pair of angles that are across from each other at the point where two lines intersect. There are two pairs of vertical angles.
The vertical intercept of a graph is the point where the graph crosses the vertical axis. If the axis is labeled with the variable y, the vertical intercept is also called the y-intercept. Also, the term is sometimes used to mean just the y-coordinate of the point where the graph crosses the vertical axis. The vertical intercept of the graph of y = 3x - 5 is (0,\text-5), or just -5.