Lesson 5: The Size of the Scale Factor

Let’s look at the effects of different scale factors.

5.1: Number Talk: Missing Factor

Solve each equation mentally.

$16x=176$

$16x=8$

$16x=1$

$\frac15x=1$

$\frac25x=1$

5.2: Scaled Copies Card Sort

Your teacher will give you a set of cards. On each card, Figure A is the original and Figure B is a scaled copy.

  1. Sort the cards based on their scale factors. Be prepared to explain your reasoning.

  2. Examine cards 10 and 13 more closely. What do you notice about the shapes and sizes of the figures? What do you notice about the scale factors?

  3. Examine cards 8 and 12 more closely. What do you notice about the figures? What do you notice about the scale factors?

5.3: Scaling A Puzzle

Your teacher will give you 2 pieces of a 6-piece puzzle.

  1. If you drew scaled copies of your puzzle pieces using a scale factor
    of $\frac12$, would they be larger or smaller than the original pieces? How do you know?
  2. Create a scaled copy of each puzzle piece on a blank square, with a scale factor of $\frac12$.
  3. When everyone in your group is finished, put all 6 of the original puzzle pieces together like this:
    A grid with 2 rows, 3 columns. First row is 1, 2, 3, and second row is 4, 5, 6.
    Next, put all 6 of your scaled copies together. Compare your scaled puzzle with the original puzzle. Which parts seem to be scaled correctly and which seem off? What might have caused those parts to be off?
  4. Revise any of the scaled copies that may have been drawn incorrectly.
  5. If you were to lose one of the pieces of the original puzzle, but still had the scaled copy, how could you recreate the lost piece?

5.4: Missing Figure, Factor, or Copy

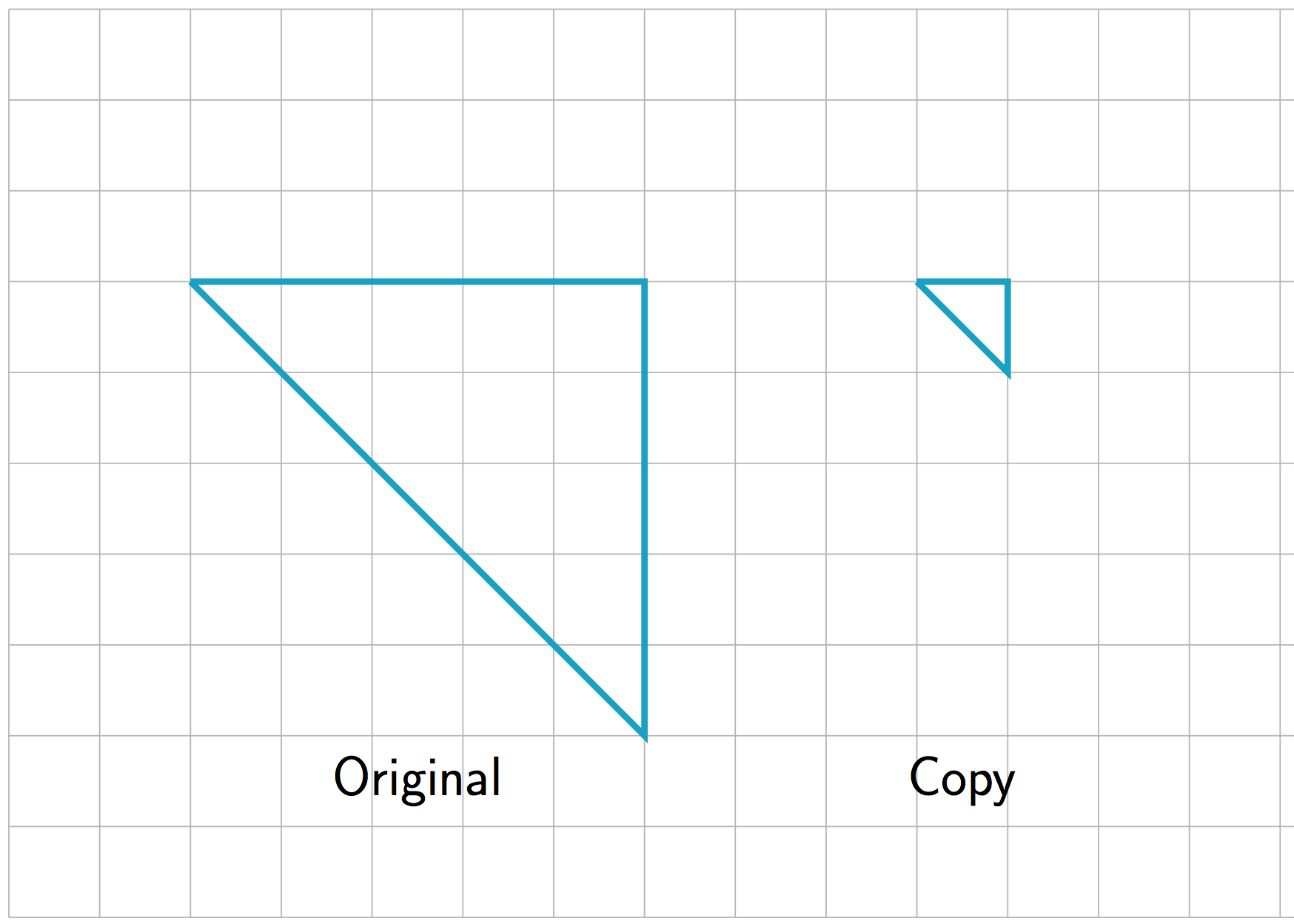
  1. What is the scale factor from the original triangle to its copy? Explain or show your reasoning.

    ​On a grid. Original triangle has sides of length 5, 5, and a diagonal length of down 5, over 5. The copy has side lengths of 1, 1, and a diagonal length of down 1, over 1.

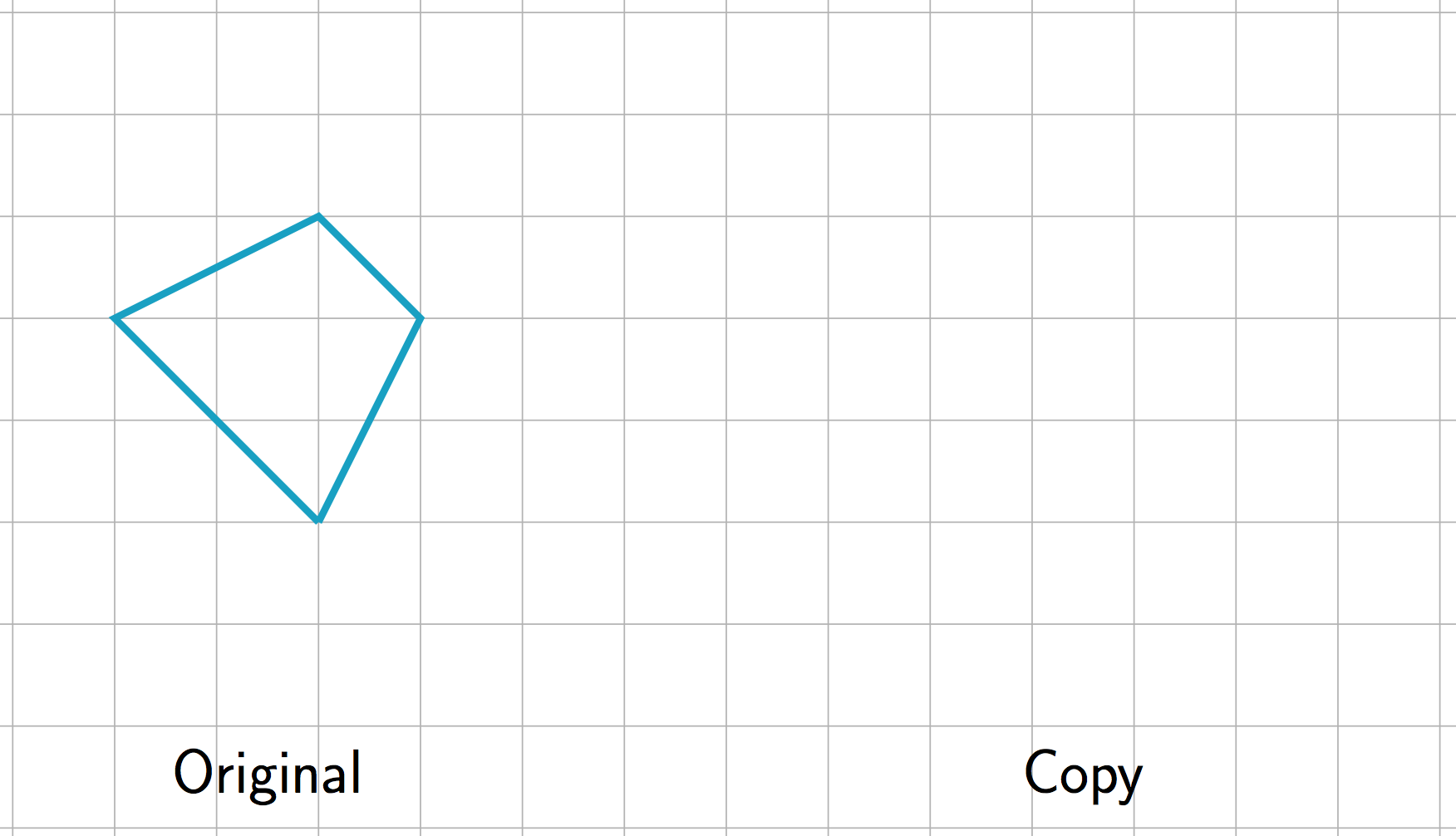
  2. The scale factor from the original trapezoid to its copy is 2. Draw the scaled copy.

    All side lengths of original figure are diagonal on the grid.Top is down 1, right 1.Right side is down 2, left 1. Bottom is left 2, up 2. Left side is up 1, right 2. Copy needs to be drawn.
  3. The scale factor from the original figure to its copy is $\frac32$. Draw the original figure.
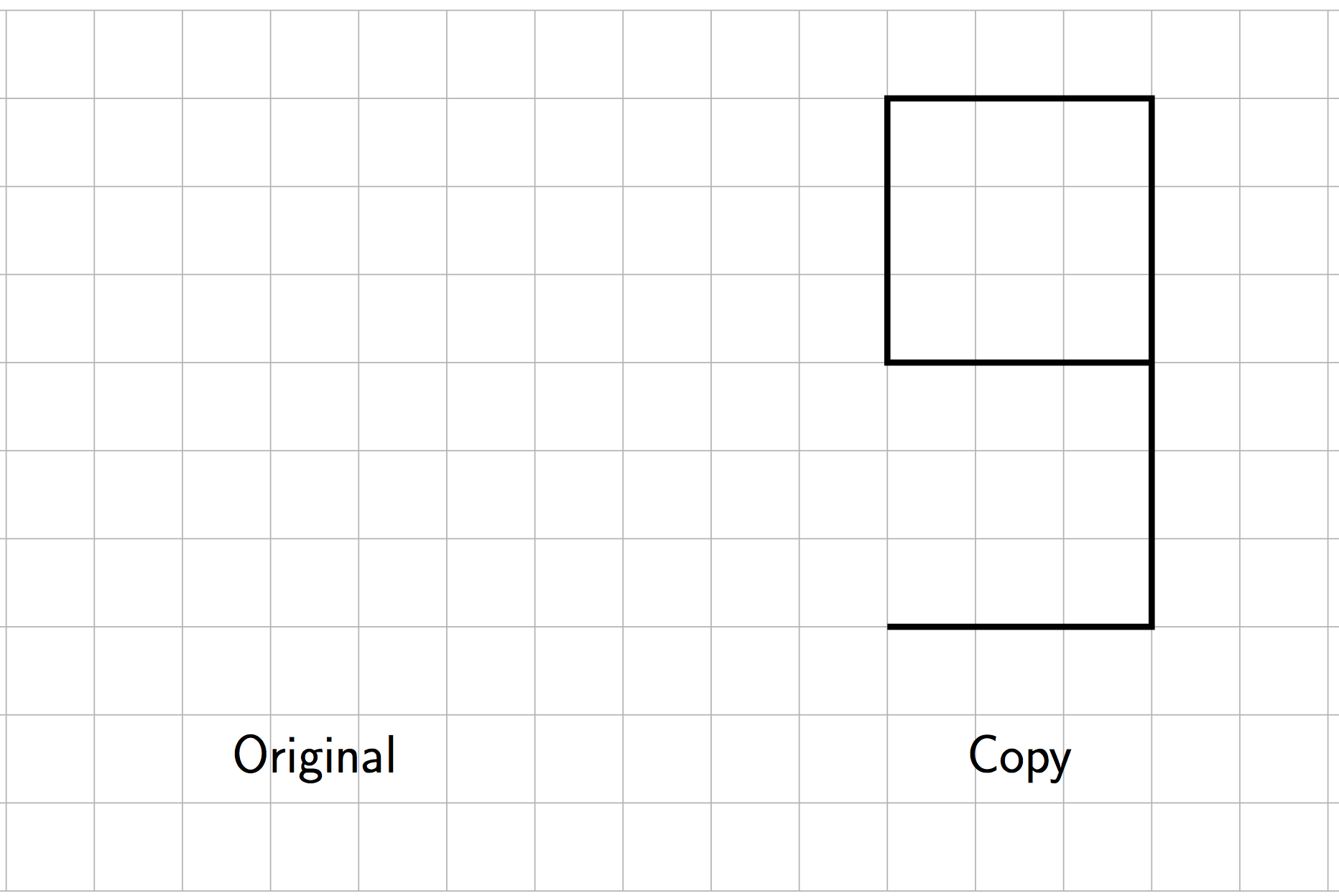
    Original needs to be drawn. The copy is on a grid and shaped like a 9 with an overall height of 6. The top of the 9 is the outline of a 3 by 3 box. The bottom horizontal is 3 units.
  4. What is the scale factor from the original figure to the copy? Explain how you know.
    the first line segment starts at the top left of the grid, slants downward 2 squares and to the right one square; the second line segment begins where the first ends, slants upward 2 squares and to the right 1 square; the third line segment beins where the second ends, slants downward 4 squares and to the right 2 squares; the fourth line segment begins where the third ends, slants upward 4 squares and to the right 2 squares.
  5. The scale factor from the original figure to its scaled copy is 3. Draw the scaled copy.
    A triangle with the horizontal side extended to the right and the vertical side extended upward creating a figure that resembles the number 4. The figure is labeled original. On the right, the position labeled copy is blank.

Summary

The size of the scale factor affects the size of the copy. When a figure is scaled by a scale factor greater than 1, the copy is larger than the original. When the scale factor is less than 1, the copy is smaller. When the scale factor is exactly 1, the copy is the same size as the original.

Triangle $DEF$ is a larger scaled copy of triangle $ABC$, because the scale factor from $ABC$ to $DEF$ is $\frac32$. Triangle $ABC$ is a smaller scaled copy of triangle $DEF$, because the scale factor from $DEF$ to $ABC$ is $\frac23$.

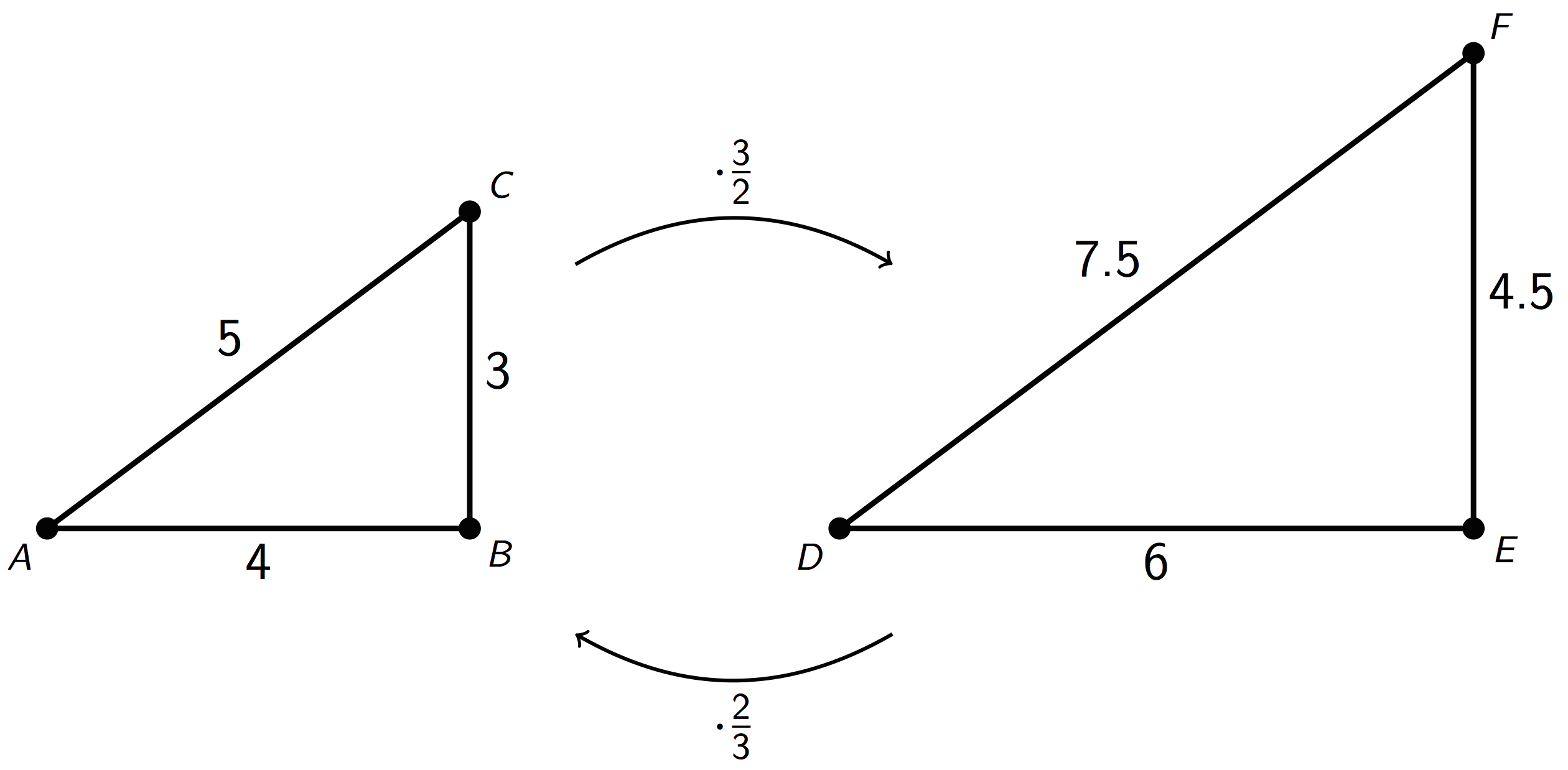
Two triangles; one labeled A B C with horizontal A B and the other D E F with horizontal D E. The length of A B is labeled 4. The length of B C is labeled 3. The length of C A is labeled 5. The length of D E is labeled 6. The length of E F is labeled 4.5. The length of F D is labeled 7.5. An arrow from triangle A B C pointing to triangle D E F is labeled, times 3 halves. An arrow from triangle D E F pointing to triangle A B C is labeled times 2 thirds.

This means that triangles $ABC$ and $DEF$ are scaled copies of each other. It also shows that scaling can be reversed using reciprocal scale factors, such as $\frac23$ and $\frac32$.

In other words, if we scale Figure A using a scale factor of 4 to create Figure B, we can scale Figure B using the reciprocal scale factor, $\frac14$, to create Figure A.

Practice Problems ▶