Lesson 13: What Makes a Good Sample?

Let’s see what makes a good sample.

13.1: Number Talk: Division by Powers of 10

Find the value of each quotient mentally.

$34,\!000\div10$

$340\div100$

$34\div10$

$3.4\div100$

13.2: Selling Paintings

Your teacher will assign you to work with either means or medians.

  1. A young artist has sold 10 paintings. Calculate the measure of center you were assigned for each of these samples:

    1. The first two paintings she sold were for \$50 and \$350.
    2. At a gallery show, she sold three paintings for \$250, \$400, and \$1,200.
    3. Her oil paintings have sold for \$410, \$400, and \$375.
  2. Here are the selling prices for all 10 of her paintings:
    \$50 \$200 \$250 \$275 \$280 \$350 \$375 \$400 \$410 \$1,200
    Calculate the measure of center you were assigned for all of the selling prices.
  3. Compare your answers with your partner. Were the measures of center for any of the samples close to the same measure of center for the population?

13.3: Sampling the Fish Market

The price per pound of catfish at a fish market was recorded for 100 weeks.

  1. What do you notice about the data from the dot plots showing the population and each of the samples within that population? What do you wonder?
  2. If the goal is to have the sample represent the population, which of the samples would be good? Which would be bad? Explain your reasoning.

GeoGebra Applet MMKbum8v

13.4: Auditing Sales

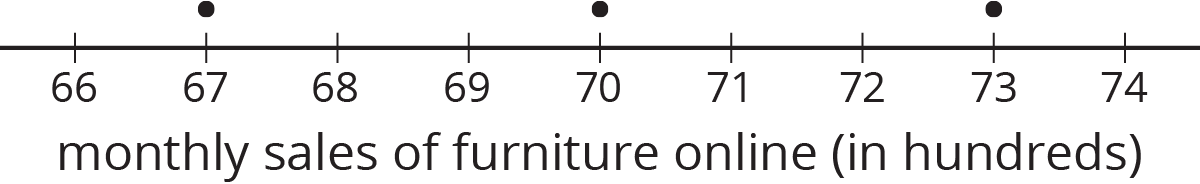
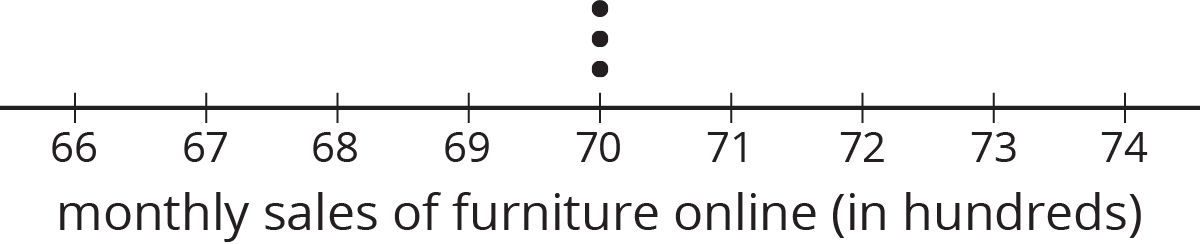
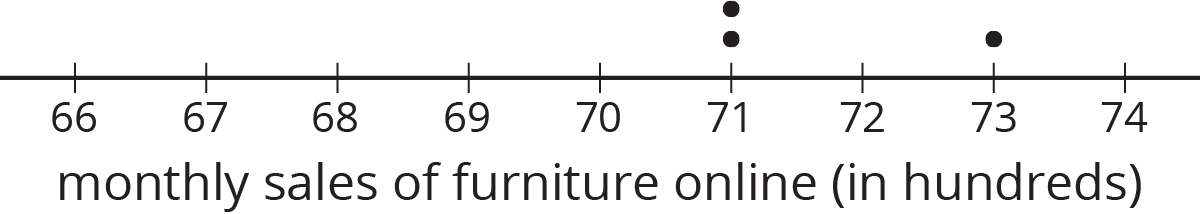
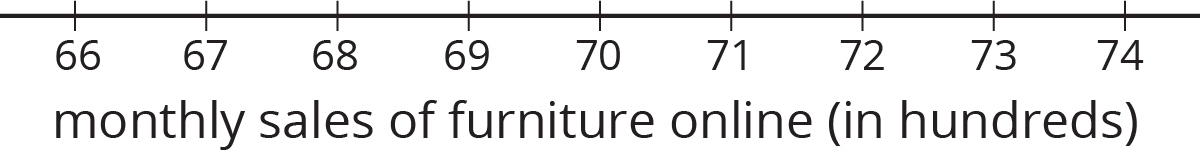
An online shopping company tracks how many items they sell in different categories during each month for a year. Three different auditors each take samples from that data. Use the samples to draw dot plots of what the population data might look like for the furniture and electronics categories.

Auditor 1's sample

A dot plot for “monthly sales of furniture online in hundreds.” The numbers 66 through 74 are indicated. The data titled "Auditor ones sample" are as follows: 67 hundred, 1 dot. 70 hundred, 1 dot. 73 hundred, 1 dot.

Auditor 2's sample

A dot plot for “monthly sales of furniture online in hundreds.” The numbers 66 through 74 are indicated. The data titled "Auditor two's sample" are as follows: 70 hundred, 3 dots.

Auditor 3's sample

A dot plot for “monthly sales of furniture online in hundreds.” The numbers 66 through 74 are indicated. The data titlted "Auditor three's sample" are as follows: 71 hundred, 2 dots. 73 hundred, 1 dot.

Population

A blank number line for “monthly sales of furniture online in hundreds.” The numbers 66 through 74 are indicated.

Auditor 1's sample

A dot plot for “monthly sales of electronics online in thousands.” The numbers 38 through 43 are indicated. The data titled "Auditor ones sample" are as follows: 39 thousand, 1 dot. 41 thousand, 1 dot. 43 thousand, 1 dot.

Auditor 2's sample

A dot plot for “monthly sales of electronics online in thousands.” The numbers 38 through 43 are indicated. The data titled "Auditor two's sample" are as follows: 41 thousand, 1 dot. 43 thousand, 2 dots.

Auditor 3's sample

A dot plot for “monthly sales of electronics online in thousands.” The numbers 38 through 43 are indicated. The data titled "Auditor three's sample" are as follows: 40 thousand, 1 dot. 41 thousand, 1 dot. 43 thousand, 1 dot.

Population

A blank number line for “monthly sales of electronics online in thousands.” The numbers 38 through 43 are indicated.

Summary

A sample that is representative of a population has a distribution that closely resembles the distribution of the population in shape, center, and spread.

For example, consider the distribution of plant heights, in cm, for a population of plants shown in this dot plot. The mean for this population is 4.9 cm, and the MAD is 2.6 cm.

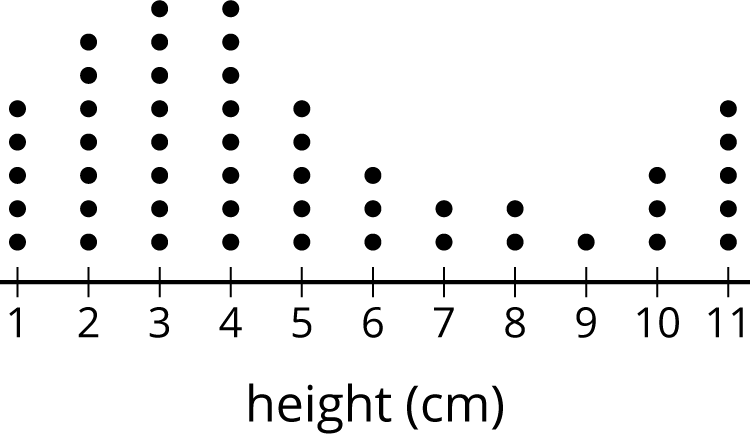
A dot plot for “height in centimeters.” The numbers 1 through 11 are indicated. The data are as follows: 1 centimeter, 5 dots; 2 centimeters, 7 dots; 3 centimeters, 8 dots; 4 centimeters, 8 dots; 5 centimeters, 5 dots; 6 centimeters, 3 dots; 7 centimeters, 2 dots; 8 centimeters, 2 dots; 9 centimeters, 1 dot; 10 centimeters, 3 dots; 11 centimeters, 5 dots.

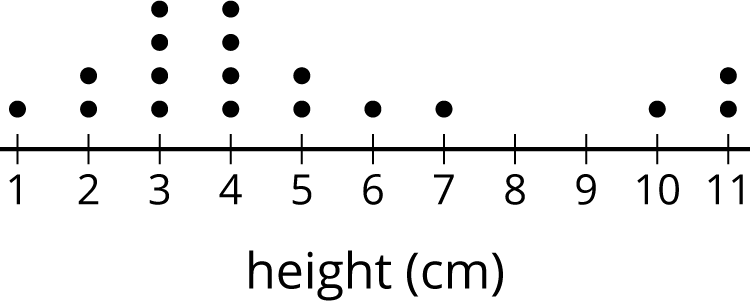
A representative sample of this population should have a larger peak on the left and a smaller one on the right, like this one. The mean for this sample is 4.9 cm, and the MAD is 2.3 cm.

A dot plot for “height in centimeters.” The numbers 1 through 11 are indicated. The data are as follows: 1 centimeter, 1 dot; 2 centimeters, 2 dots; 3 centimeters, 4 dots; 4 centimeters, 4 dots; 5 centimeters, 2 dots; 6 centimeters, 1 dot; 7 centimeters, 1 dot; 10 centimeters, 1 dot; 11 centimeters, 2 dots.

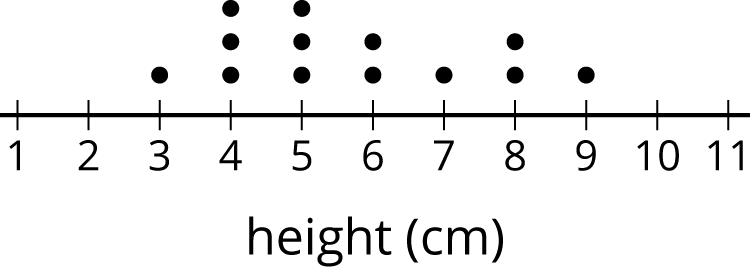
Here is the distribution for another sample from the same population. This sample has a mean of 5.7 cm and a MAD of 1.5 cm. These are both very different from the population, and the distribution has a very different shape, so it is not a representative sample.

A dot plot for “height in centimeters.” The numbers 1 through 11 are indicated. The data are as follows: 3 centimeters, 1 dot; 4 centimeters, 3 dots; 5 centimeters, 3 dots; 6 centimeters, 2 dots; 7 centimeters, 1 dot; 8 centimeters, 2 dots; 9 centimeters, 1 dot.

Practice Problems ▶

Glossary

representative

representative

A sample that is representative of a population has a distribution that closely resembles the distribution of the population in shape, center, and spread.