Lesson 7: Adding and Subtracting to Solve Problems

Let’s apply what we know about signed numbers to different situations.

7.1: Positive or Negative?

Without computing:

  1. Is the solution to $\text-2.7 + x = \text- 3.5$ positive or negative?

  2. Which of the following are solutions to $\text-2.7 + x = \text- 3.5$?

    $\text-3.5 + 2.7$

    $3.5 - 2.7$

    $\text-3.5 - (\text-2.7)$

    $\text-3.5 - 2.7$

7.2: Phone Inventory

A store tracks the number of cell phones it has in stock and how many phones it sells. The table shows the inventory for one phone model at the beginning of each day last week. The inventory changes when they sell phones or get shipments of phones into the store.

  inventory change
Monday 18 -2
Tuesday 16 -5
Wednesday 11 -7
Thursday 4 -6
Friday -2 20
  1. What do you think it means when the change is positive? Negative?
  2. What do you think it means when the inventory is positive? Negative?
  3. Based on the information in the table, what do you think the inventory will be at on Saturday morning? Explain your reasoning.
  4. What is the difference between the greatest inventory and the least inventory?

7.3: Solar Power

Han's family got a solar panel. Each month they get a credit to their account for the electricity that is generated by the solar panel. The credit they receive varies based on how sunny it is.

Solar Panels on a Roof Copyright Owner: Pujanak (Own work) License: Public Domain Via: Wikimedia Commons

In January they used \$83.56 worth of electricity and generated \$6.75 worth of electricity. Here is their electricity bill from January.

Current charges: \$83.56

Solar Credit: -\$6.75

Amount due: \$74.81

  1. In July they were traveling away from home and only used \$19.24 worth of electricity. Their solar panel generated \$22.75 worth of electricity. What was their amount due in July?

  2. The table shows the value of the electricity they used and the value of the electricity they generated each week for a month. What amount is due for this month?

      used (\$) generated (\$)
    week 1 13.45 -6.33
    week 2 21.78 -8.94
    week 3 18.12 -7.70
    week 4 24.05 -5.36

     

  3. What is the difference between the value of the electricity generated in week 1 and week 2? Between week 2 and week 3?

7.4: Differences and Distances

Plot these points on the coordinate grid: $A= (5, 4), B= (5, \text-2), C= (\text-3, \text-2), D= (\text-3, 4)$

GeoGebra Applet yNJ6guSn

  1. What shape is made if you connect the dots in order?
  2. What are the side lengths of figure $ABCD$?
  3. What is the difference between the $x$-coordinates of $B$ and $C$?
  4. What is the difference between the $x$-coordinates of $C$ and $B$?
  5. How do the differences of the coordinates relate to the distances between the points?

Summary

Sometimes we use positive and negative numbers to represent quantities in context. Here are some contexts we have studied that can be represented with positive and negative numbers:

  • temperature
  • elevation
  • inventory
  • an account balance
  • electricity flowing in and flowing out

In these situations, using positive and negative numbers, and operations on positive and negative numbers, helps us understand and analyze them. To solve problems in these situations, we just have to understand what it means when the quantity is positive, when it is negative, and what it means to add and subtract them.

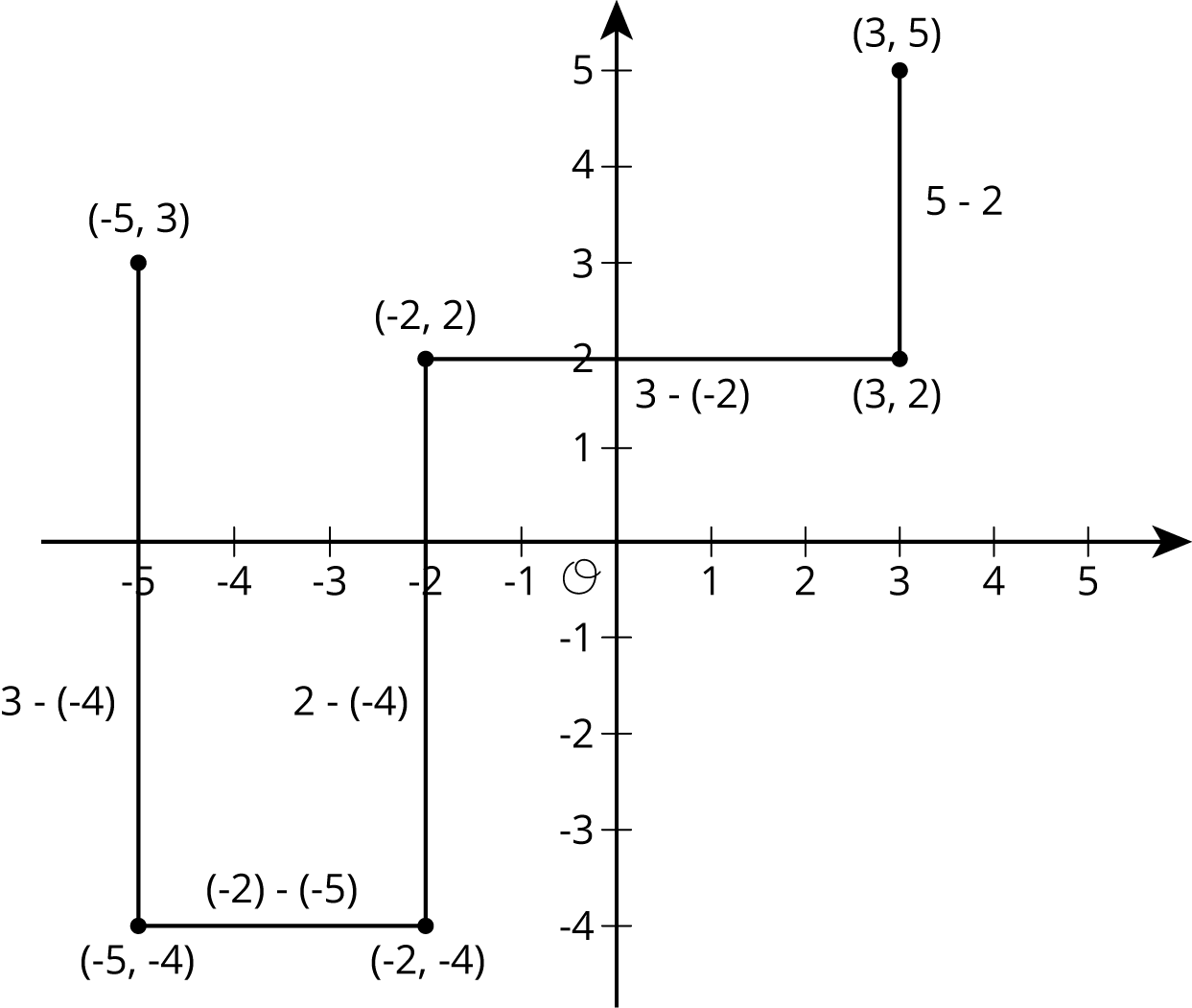
When two points in the coordinate plane lie on a horizontal line, you can find the distance between them by subtracting their $x$-coordinates.

When two points in the coordinate plane lie on a horizontal line, you can find the distance between them by subtracting their $y$-coordinates.

Five line segments on a coordinate plane with the origin labeled “O”. The numbers negative 5 through 5, are indicated on the horizontal axis and the numbers negative 4 through 5 are indicated on the vertical axis. Each line segment is either vertical or horizontal. The first line segment is vertical that begins at the point negative 5 comma 3 and ends at the point negative 5 comma negative 4; the line segment is labeled with the expression 3 minus negative 4. The second line segment is horizontal and begins at the point negative 5 comma negative 4 and ends at the point negative 2 comma negative 4; the line segment is labeled with the expression negative 2 minus negative 5. The third line segment is vertical and begins at the point negative 2 comma negative 4 and ends at the point negative 2 comma 2; the line segment is labeled with the expression 2 minus negative 4. The fourth line segment is horizontal and begins at the point negative 2 comma 2 and ends at the point three comma 2; the line segment is labeled with the expression 3 minus negative 2. The fifth line segment is vertical and begins at the point 3 comma 2 and ends at the point 3 comma five; the line segment is labeled with the expression 5 minus 2.

Remember: the distance between two numbers is independent of the order, but the difference depends on the order.

Practice Problems ▶