Lesson 8: Scale Drawings and Maps

Let’s use scale drawings to solve problems.

8.1: A Train and a Car

Two cities are 243 miles apart.

  • It takes a train 4 hours to travel between the two cities at a constant speed.

  • A car travels between the two cities at a constant speed of 65 miles per hour.

Which is traveling faster, the car or the train? Be prepared to explain your reasoning.

8.2: Driving on I-90

  1. A driver is traveling at a constant speed on Interstate 90 outside of Chicago. If she traveled from Point A to Point B in 8 minutes, did she obey the speed limit of 55 miles per hour? Explain your reasoning.
  2. A traffic helicopter flew directly from Point A to Point B in 8 minutes. Did the helicopter travel faster or slower than the driver? Explain or show your reasoning.

Use the Distance tool An image of the distance tool. to measure the shortest distance between two points.

GeoGebra Applet aVkbzxQ7

8.3: Biking through Kansas

A cyclist rides at a constant speed of 15 miles per hour. At this speed, about how long would it take the cyclist to ride from Garden City to Dodge City, Kansas?

Cyclist photo Copyright Owner: skeeze License: Public Domain Via: Pixabay

Map of Kansas Copyright Owner: American Fact Finder by the United States Census Bureau License: Public Domain Via: American Fact Finder

Summary

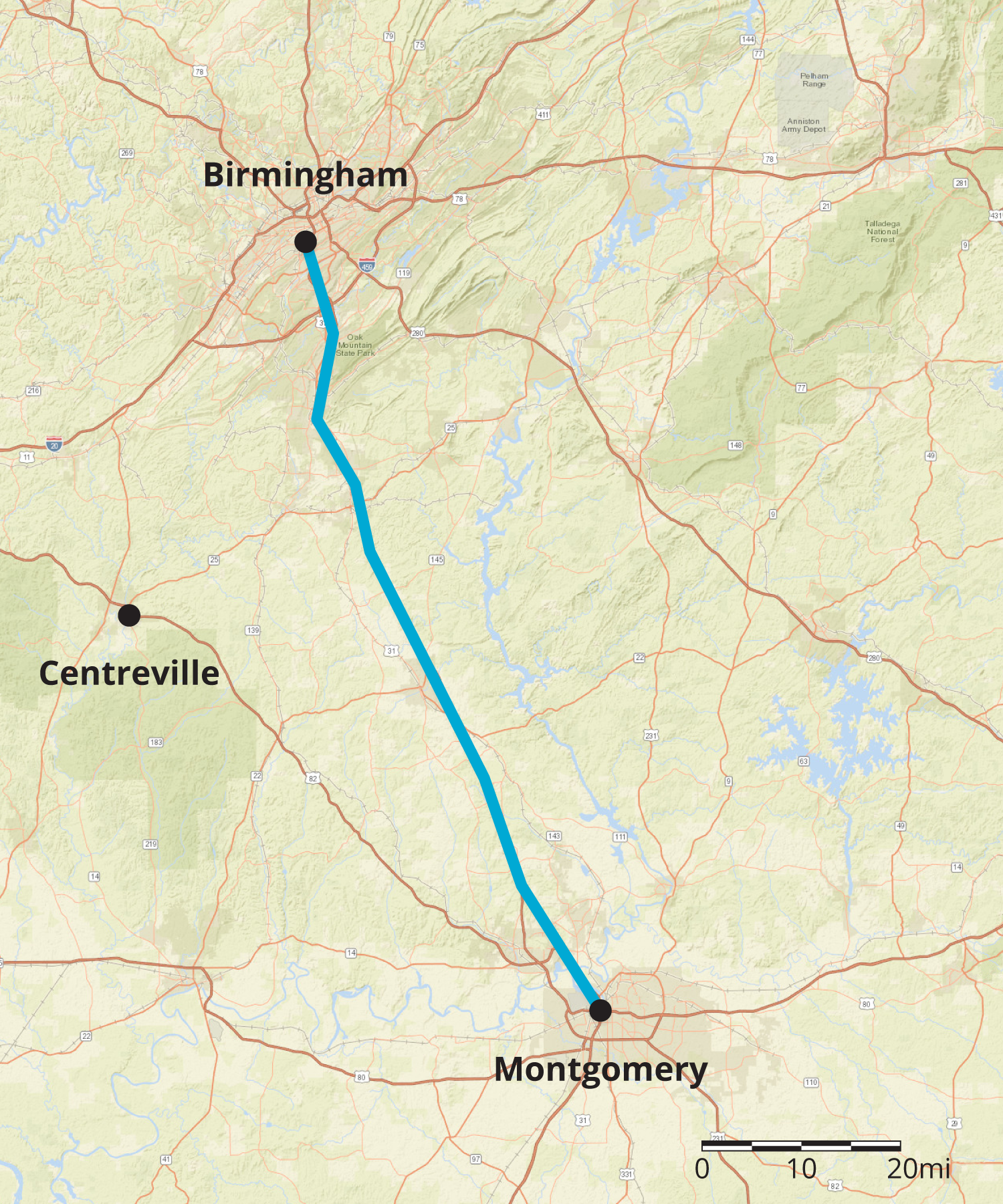
Maps with scales are useful for making calculations involving speed, time, and distance. Here is a map of part of Alabama.

A map of a part of Alabama. Three points indicate three different cities on the map. The bottom point is labeled Montgomery, the middle point is labeled Centreville, and the top point is labeled Birmingham. A scale on the map shows 1 inch equals 20 miles. The distance between Birmingham and Montgomery is approximately 4 point 5 inches on the map.

Suppose it takes a car 1 hour and 30 minutes to travel at constant speed from Birmingham to Montgomery. How fast is the car traveling?

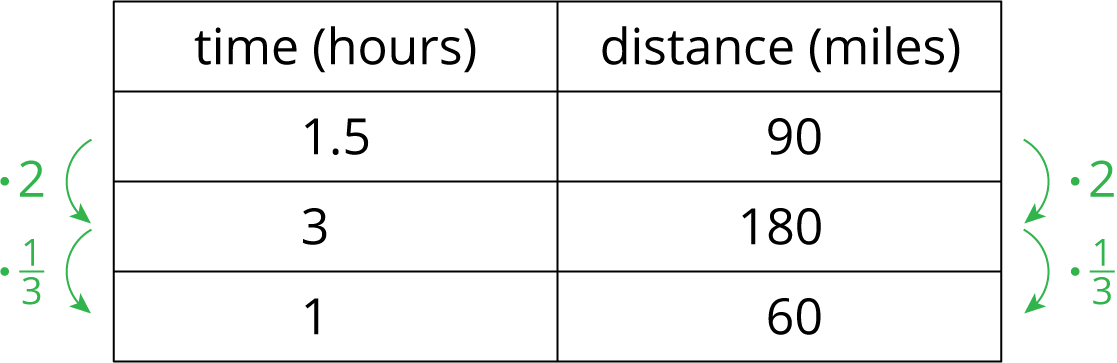
To make an estimate, we need to know about how far it is from Birmingham to Montgomery. The scale of the map represents 20 miles, so we can estimate the distance between these cities is about 90 miles.

Since 90 miles in 1.5 hours is the same speed as 180 miles in 3 hours, the car is traveling about 60 miles per hour.

Suppose a car is traveling at a constant speed of 60 miles per hour from Montgomery to Centreville. How long will it take the car to make the trip? Using the scale, we can estimate that it is about 70 miles. Since 60 miles per hour is the same as 1 mile per minute, it will take the car about 70 minutes (or 1 hour and 10 minutes) to make this trip.

Practice Problems ▶