When two different situations can be described by equivalent ratios, that means they are alike in some important way.
An example is a recipe. If two people make something to eat or drink, the taste will only be the same as long as the ratios of the ingredients are equivalent. For example, all of the mixtures of water and drink mix in this table taste the same, because the ratios of cups of water to scoops of drink mix are all equivalent ratios.
| row 1 |
water (cups) |
drink mix (scoops) |
| row 2 |
3 |
1 |
| row 3 |
12 |
4 |
| row 4 |
1.5 |
0.5 |
If a mixture were not equivalent to these, for example, if the ratio of cups of water to scoops of drink mix were $6:4$, then the mixture would taste different.
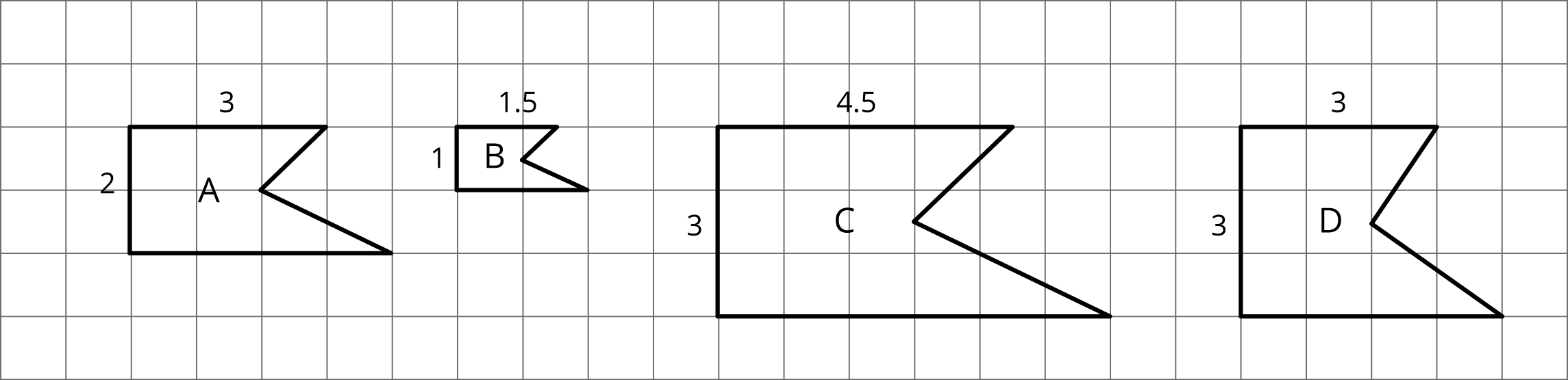
Notice that the ratios of pairs of corresponding side lengths are equivalent in figures A, B, and C. For example, the ratios of the length of the top side to the length of the left side for figures A, B, and C are equivalent ratios. Figures A, B, and C are scaled copies of each other; this is the important way in which they are alike.
If a figure has corresponding sides that are not in a ratio equivalent to these, like figure D, then it’s not a scaled copy. In this unit, you will study relationships like these that can be described by a set of equivalent ratios.