11.1: Remember Tape Diagrams
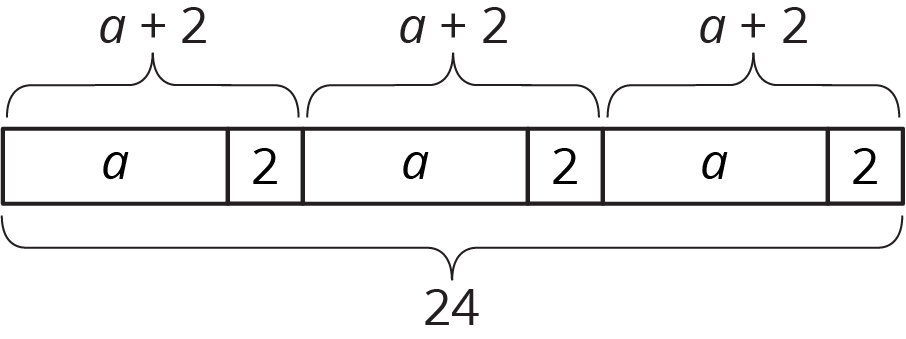
- Write a story that could be represented by this tape diagram.
- Write an equation that could be represented by this tape diagram.
Let’s use tape diagrams, equations, and reasoning to solve problems.


Explain how each part of the situation is represented in Tyler’s diagram:
How many total invitations Tyler is trying to make.
How many invitations he has made already.
How many days he has to finish the invitations.
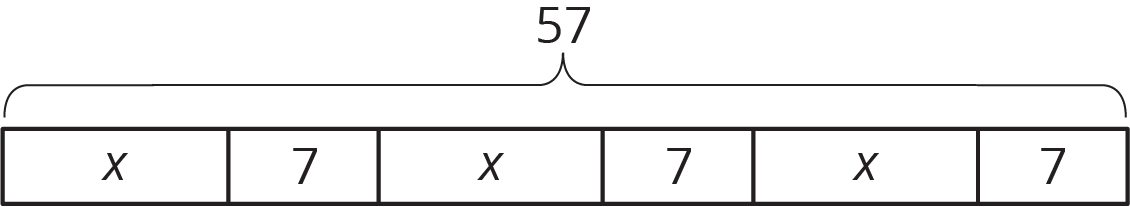
Noah and his sister are making prize bags for a game at the fair. Noah is putting 7 pencil erasers in each bag. His sister is putting in some number of stickers. After filling 3 of the bags, they have used a total of 57 items.
Priya, Han, and Elena, are members of the running club at school.
Priya was busy studying this week and ran 7 fewer miles than last week. She ran 9 times as far as Elena ran this week. Elena only had time to run 4 miles this week.
One day last week, 6 teachers joined \frac57 of the members of the running club in an after-school run. Priya counted a total of 31 people running that day. How many members does the running club have?
Priya and Han plan a fundraiser for the running club. They begin with a balance of \text-80 because of expenses. In the first hour of the fundraiser they collect equal donations from 9 parents, which brings their balance to \text-44. How much did each parent give?
The running club uses the money they raised to pay for a trip to a canyon. At one point during a run in the canyon, the students are at an elevation of 128 feet. After descending at a rate of 50 feet per minute, they reach an elevation of \text-472 feet. How long did the descent take?
A musician performed at three local fairs. At the first he doubled his money and spent $30. At the second he tripled his money and spent $54. At the third, he quadrupled his money and spent $72. In the end he had $48 left. How much did he have before performing at the fairs?
Many problems can be solved by writing and solving an equation. Here is an example:
Clare ran 4 miles on Monday. Then for the next six days, she ran the same distance each day. She ran a total of 22 miles during the week. How many miles did she run on each of the 6 days?
One way to solve the problem is to represent the situation with an equation, 4+6x = 22, where x represents the distance, in miles, she ran on each of the 6 days. Solving the equation gives the solution to this problem.
\begin{align} 4+6x &= 22 \\ 6x &= 18 \\ x &= 3 \\ \end{align}