8.1: From 100 to 106
How do you get from one number to the next using multiplication or division?
- From 100 to 106
- From 100 to 90
- From 90 to 100
- From 106 to 100
Let’s use equations to represent increases and decreases.
How do you get from one number to the next using multiplication or division?
The value of a new car decreases by about 15% in the first year. How much will a car be worth after one year if its initial value was \$1,000? \$5,000? \$5,020? $x$ dollars? If you get stuck, consider using diagrams or a table to organize your work.


Match an equation to each of these situations. Be prepared to share your reasoning.
$0.23x = 52$
$0.77x = 52$
$1.23x = 52$
$1.77x = 52$
An astronaut was exploring the moon of a distant planet, and found some glowing goo at the bottom of a very deep crater. She brought a 10-gram sample of the goo to her laboratory. She found that when the goo was exposed to light, the total amount of goo increased by 100% every hour.
The gas tank in dad’s car holds 12 gallons. The gas tank in mom’s truck holds 50% more than that. How much gas does the truck’s tank hold?
Explain why this situation can be represented by the equation $(1.5) \boldcdot 12 = t$. Make sure that you explain what $t$ represents.
We can use equations to express percent increase and percent decrease. For example, if $y$ is 15% more than $x$,

we can represent this using any of these equations:
$y = x + 0.15x$
$y = (1 + 0.15)x$
$y = 1.15x$
So if someone makes an investment of $x$ dollars, and its value increases by 15% to \$1250, then we can write and solve the equation $1.15x =1250$ to find the value of the initial investment.
Here is another example: if $a$ is 7% less than $b$,
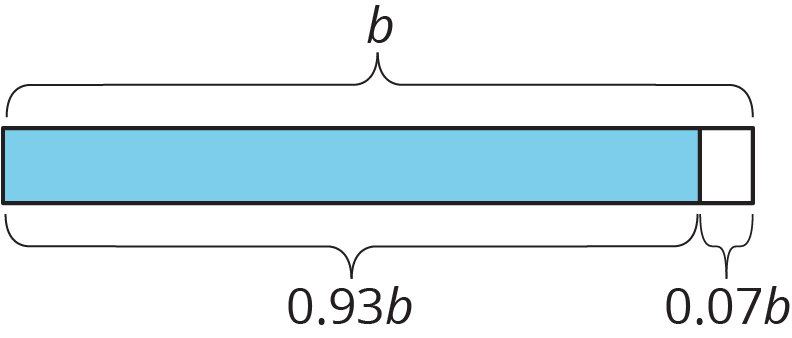
we can represent this using any of these equations:
$a = b - 0.07b$
$a = (1-0.07)b$
$a = 0.93b$
So if the amount of water in a tank decreased 7% from its starting value of $b$ to its ending value of 348 gallons, then you can write $0.93b = 348$.
Often, an equation is the most efficient way to solve a problem involving percent increase or percent decrease.