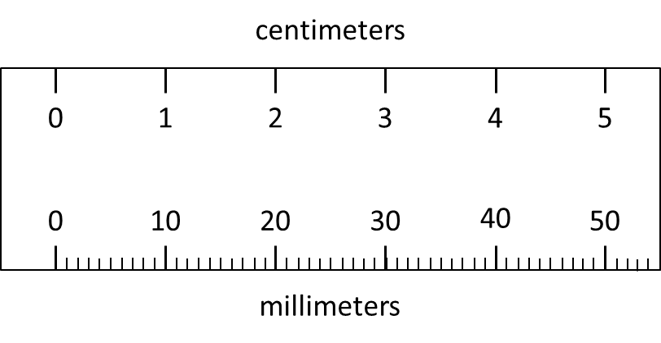
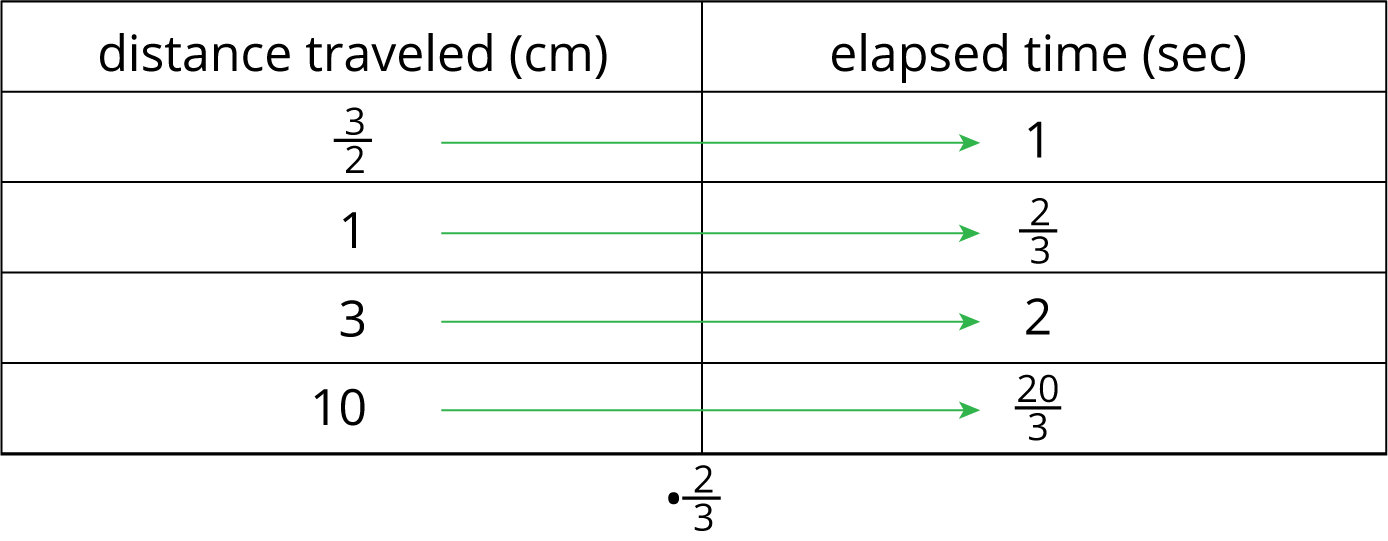
When something is traveling at a constant speed, there is a proportional relationship between the time it takes and the distance traveled. The table shows the distance traveled and elapsed time for a bug crawling on a sidewalk.
We can multiply any number in the first column by $\frac23$ to get the corresponding number in the second column. We can say that the elapsed time is proportional to the distance traveled, and the constant of proportionality is $\frac23$. This means that the bug’s pace is $\frac23$ seconds per centimeter.
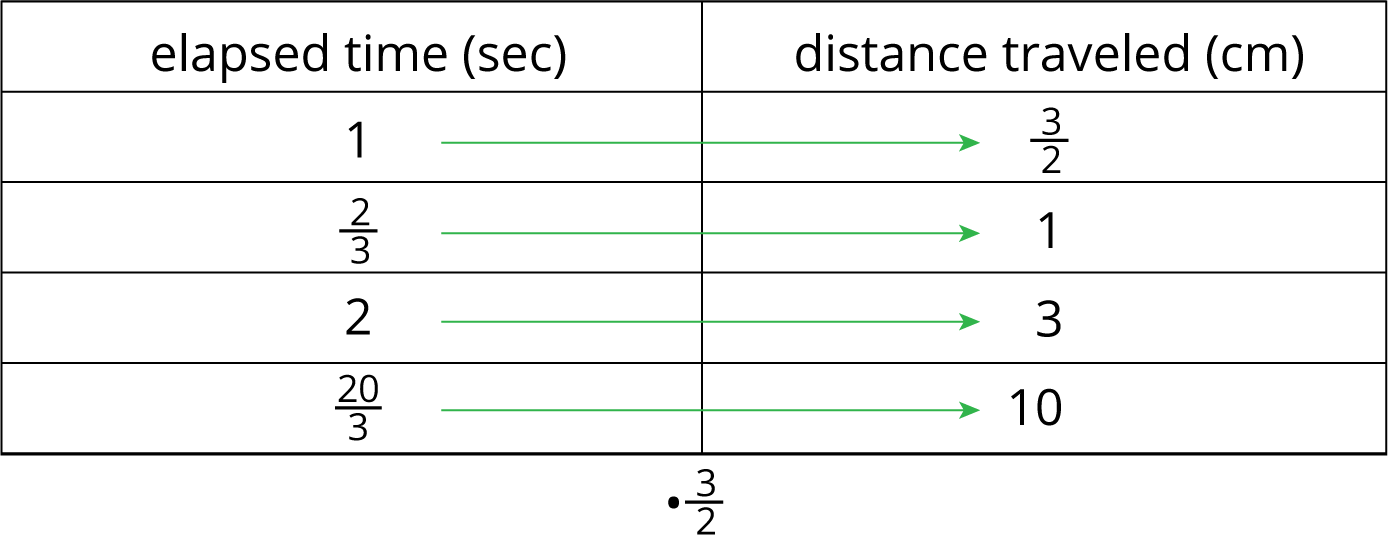
This table represents the same situation, except the columns are switched.
We can multiply any number in the first column by $\frac32$ to get the corresponding number in the second column. We can say that the distanced traveled is proportional to the elapsed time, and the constant of proportionality is $\frac32$. This means that the bug’s speed is $\frac32$ centimeters per second.
Notice that $\frac32$ is the reciprocal of $\frac23$. When two quantities are in a proportional relationship, there are two constants of proportionality, and they are always reciprocals of each other. When we represent a proportional relationship with a table, we say the quantity in the second column is proportional to the quantity in the first column, and the corresponding constant of proportionality is the number we multiply values in the first column to get the values in the second.