Let's solve problems about adding signed numbers.
Draw arrows on a number line to represents these situations:
The temperature was -5 degrees. Then the temperature rose 5 degrees.
A climber was 30 feet above sea level. Then she descended 30 feet.
What’s the opposite?
Running 150 feet east.
Jumping down 10 steps.
Pouring 8 gallons into a fish tank.
Explore the applet and then answer the questions.
A mountaineer is climbing on a cliff. She is 200 feet above the ground. If she climbs up, this will be a positive change. If she climbs down, this will be a negative change.
Complete the table.
| starting elevation (feet) | change (feet) | final elevation (feet) | |
|---|---|---|---|
| row 1 | +200 | +70 | |
| row 2 | +200 | -120 | |
| row 3 | +200 | +300 | |
| row 4 | +200 | +110 | |
| row 5 | +200 | +35.2 |
Select three rows from the table and draw a number line diagram for each. Include the starting elevation, change, and final elevation on each diagram.
A spelunker is down in a cave next to the cliff. If she climbs down deeper into the cave, this will be a negative change. If she climbs up, whether inside the cave or out of the cave and up the cliff, this will be a positive change.
Complete the table.
| starting elevation (meters) | change (meters) | final elevation (meters) | |
|---|---|---|---|
| row 1 | -20 | -15 | |
| row 2 | -20 | +130 | |
| row 3 | -20 | +61 | |
| row 4 | -20 | -5 | |
| row 5 | -20 | -50 | |
| row 6 | -20 | +27.5 | |
| row 7 | -20 | +73.5 |
Select three rows from the table and draw a number line diagram for each. Include the starting elevation, change, and final elevation on each diagram.
Complete the table and draw a number line diagram to represent each row in this table.
| starting elevation (meters) | change (meters) | final elevation (meters) | |
|---|---|---|---|
| row 1 | +200 | -200 | |
| row 2 | -20 | 0 |
Find the sums.
Find the sum without a calculator.
Your teacher will give you a long strip of paper.
Follow these instructions to create a number line.
$a$
$b$
$2a$
$2b$
$a + b$
$\text-a$
$\text-b$
$a + \text-b$
$b + \text-a$
Complete each statement using <, >, or =. Use your number line to explain your reasoning.
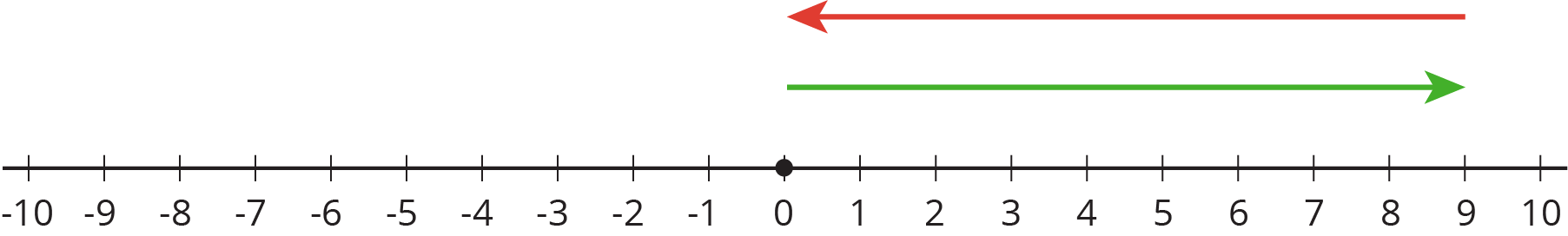
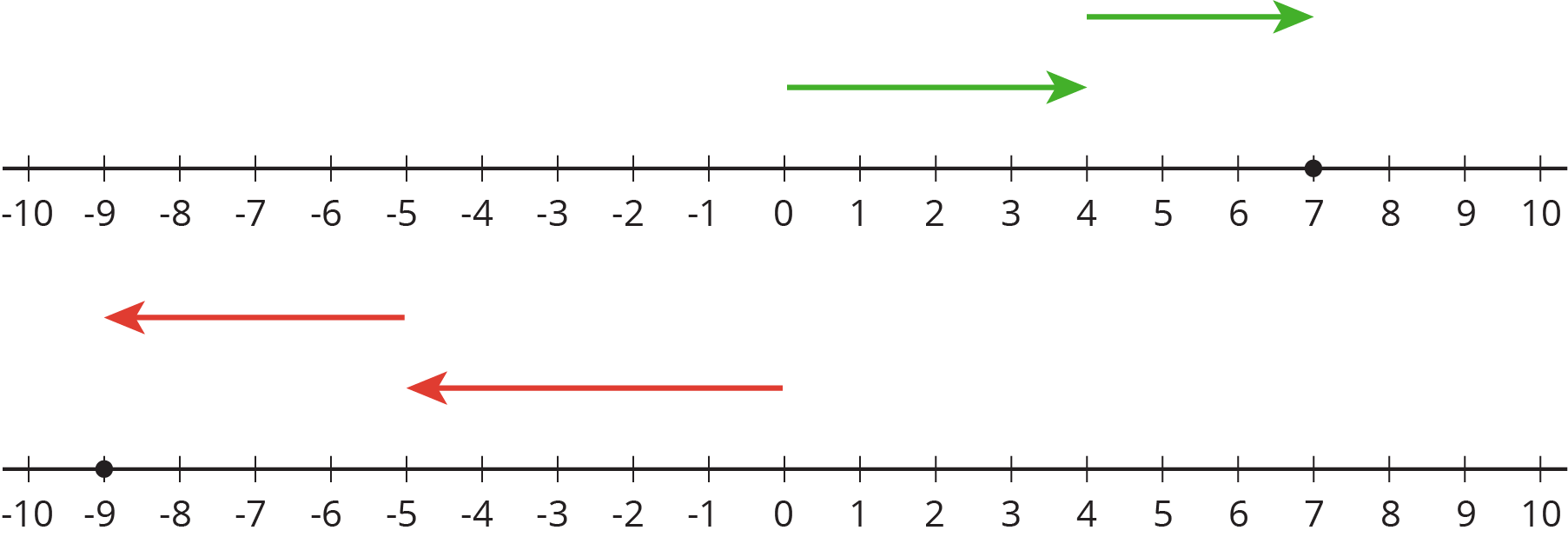
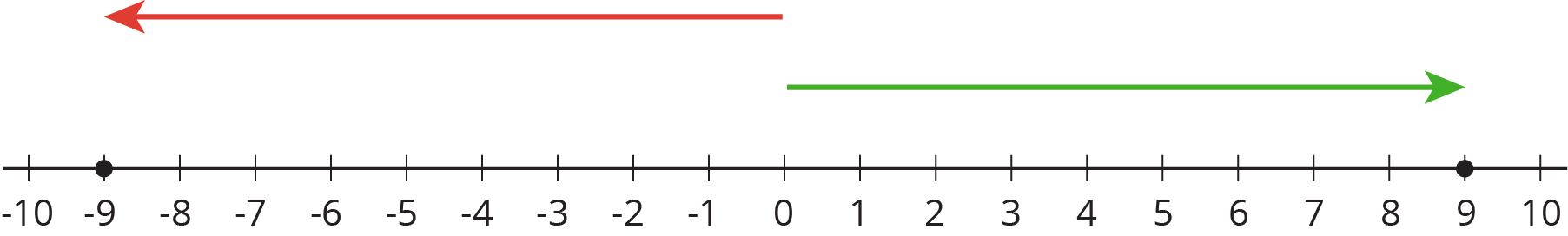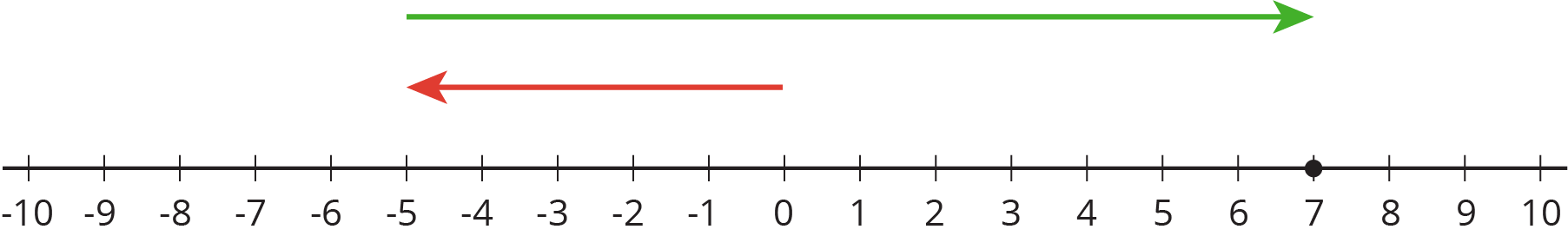The opposite of a number is the same distance from 0 but on the other side of 0.
The opposite of -9 is 9. When we add opposites, we always get 0. This diagram shows that $9 + \text-9 = 0$.
When we add two numbers with the same sign, the arrows that represent them point in the same direction. When we put the arrows tip to tail, we see the sum has the same sign.
To find the sum, we add the magnitudes and give it the correct sign. For example, $(\text-5) + (\text-4) =\text - (5 + 4)$.
On the other hand, when we add two numbers with different signs, we subtract their magnitudes (because the arrows point in the opposite direction) and give it the sign of the number with the larger magnitude. For example, $(\text-5) + 12 = +(12 - 5)$.
