Lesson 10: Introducing Graphs of Proportional Relationships

Let’s see how graphs of proportional relationships differ from graphs of other relationships.

10.1: Notice These Points

Plot the points. What do you notice about the graph?

$(0,10), (1,8), (2,6), (3,4), (4,2)$

GeoGebra Applet yA3xMKvH

10.2: T-shirts for Sale

Some T-shirts cost \$8 each.

  1. Use the table to answer these questions.

    1. What does $x$ represent?
    2. What does $y$ represent?
    3. Is there a proportional relationship between $x$ and $y$?
        $x$     $y$  
    row 1 1 8
    row 2 2 16
    row 3 3 24
    row 4 4 32
    row 5 5 40
    row 6 6 48
  2. Plot the pairs in the table on the coordinate plane. 

    GeoGebra Applet z3hY3qBZ

  3. What do you notice about the graph?

10.3: Matching Tables and Graphs

Your teacher will give you papers showing tables and graphs.

  1. Examine the graphs closely. What is the same and what is different about the graphs?
  2. Sort the graphs into categories of your choosing. Label each category. Be prepared to explain why you sorted the graphs the way you did.

  3. Take turns with a partner to match a table with a graph.

    1. For each match you find, explain to your partner how you know it is a match.
    2. For each match your partner finds, listen carefully to their explanation. If you disagree, work to reach an agreement.

    Pause here so your teacher can review your work.

  4. Trade places with another group. How are their categories the same as your group's categories? How are they different?
  5. Return to your original place. Discuss any changes you may wish to make to your categories based on what the other group did.
  6. Which of the relationships are proportional?
  7. What have you noticed about the graphs of proportional relationships? Do you think this will hold true for all graphs of proportional relationships?

Summary

One way to represent a proportional relationship is with a graph. Here is a graph that represents different amounts that fit the situation, “Blueberries cost \$6 per pound.”

Different points on the graph tell us, for example, that 2 pounds of blueberries cost \$12, and 4.5 pounds of blueberries cost \$27.

Sometimes it makes sense to connect the points with a line, and sometimes it doesn’t. We could buy, for example, 4.5 pounds of blueberries or 1.875 pounds of blueberries, so all the points in between the whole numbers make sense in the situation, so any point on the line is meaningful.

If the graph represented the cost for different numbers of sandwiches (instead of pounds of blueberries), it might not make sense to connect the points with a line, because it is often not possible to buy 4.5 sandwiches or 1.875 sandwiches. Even if only points make sense in the situation, though, sometimes we connect them with a line anyway to make the relationship easier to see.

Graphs that represent proportional relationships all have a few things in common:

  • Points that satisfy the relationship lie on a straight line.
  • The line that they lie on passes through the origin, $(0,0)$.

Here are some graphs that do not represent proportional relationships:

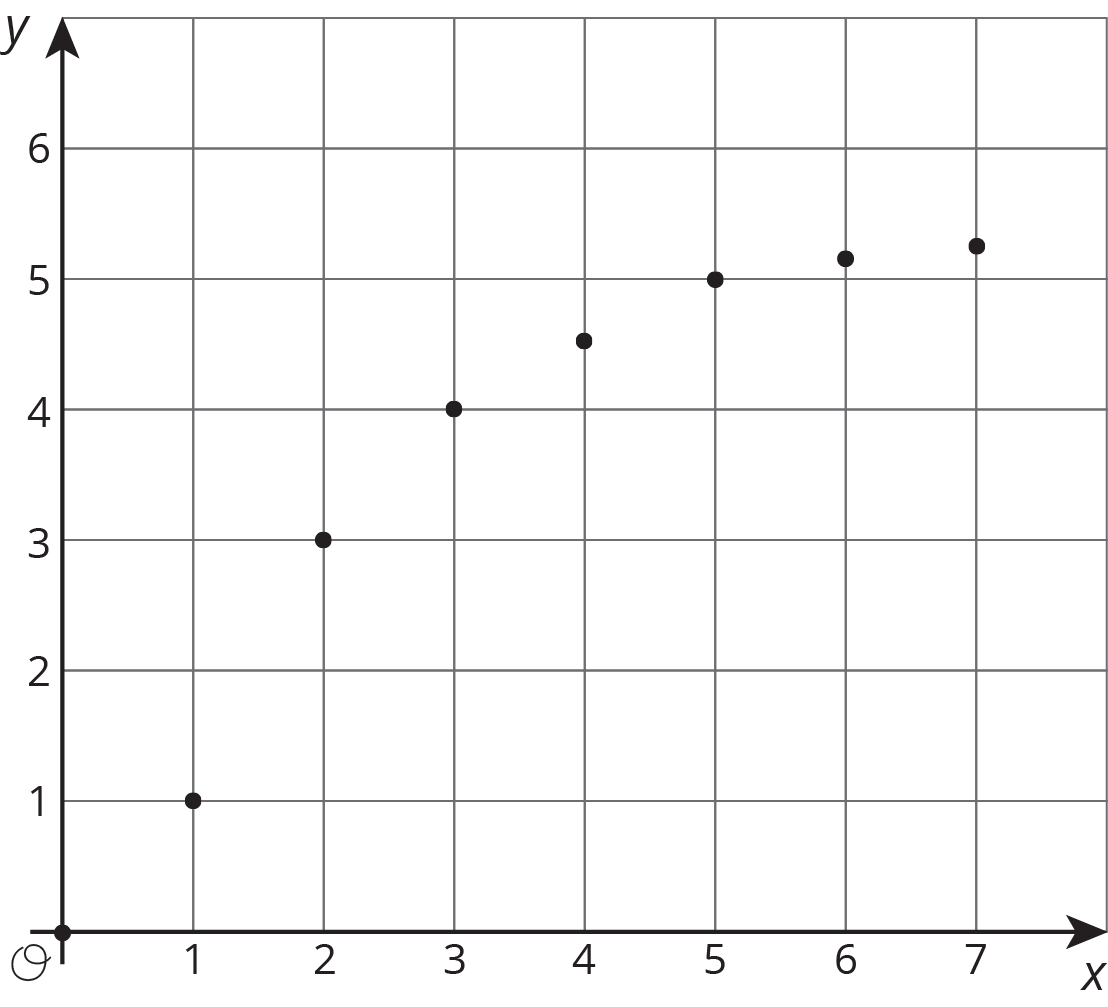
Seven points plotted in the coordinate plane with the origin labeled “O”. The x axis has the numbers 0 through 7 indicated. The y axis has the numbers 0 through 6 indicated. The points with coordinates 1 comma 1, 2 comma 3, 3 comma 4, 4 comma 4 point 5, 5 comma 5, 6 comma 5 point 1, and 7 comma 5 point 2 are indicated.

These points do not lie on a line.

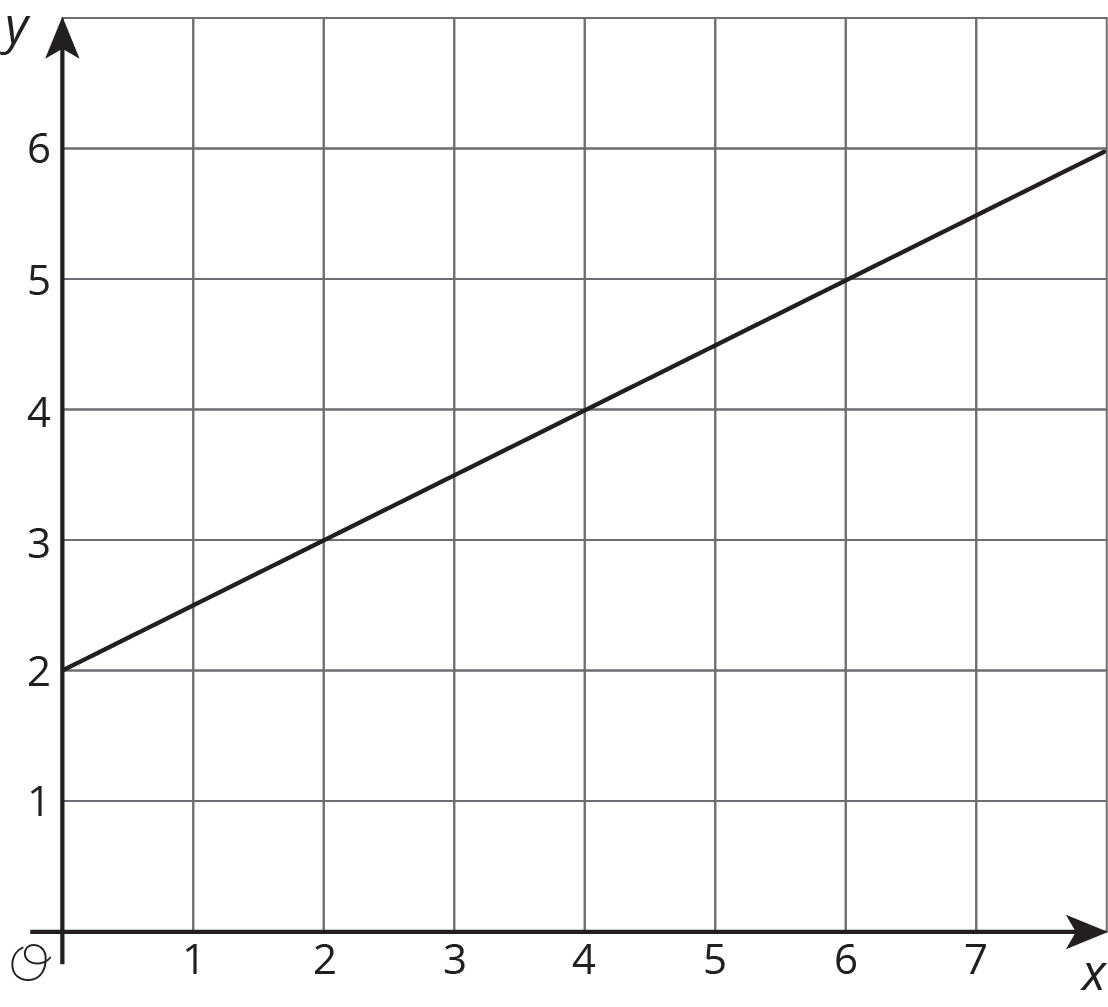
This is a line, but it doesn’t go through the origin.

 

Practice Problems ▶

Glossary

origin

origin

In the coordinate plane, the origin is the point $(0,0)$.