Lesson 6: Scaling and Area

Let's build scaled shapes and investigate their areas.

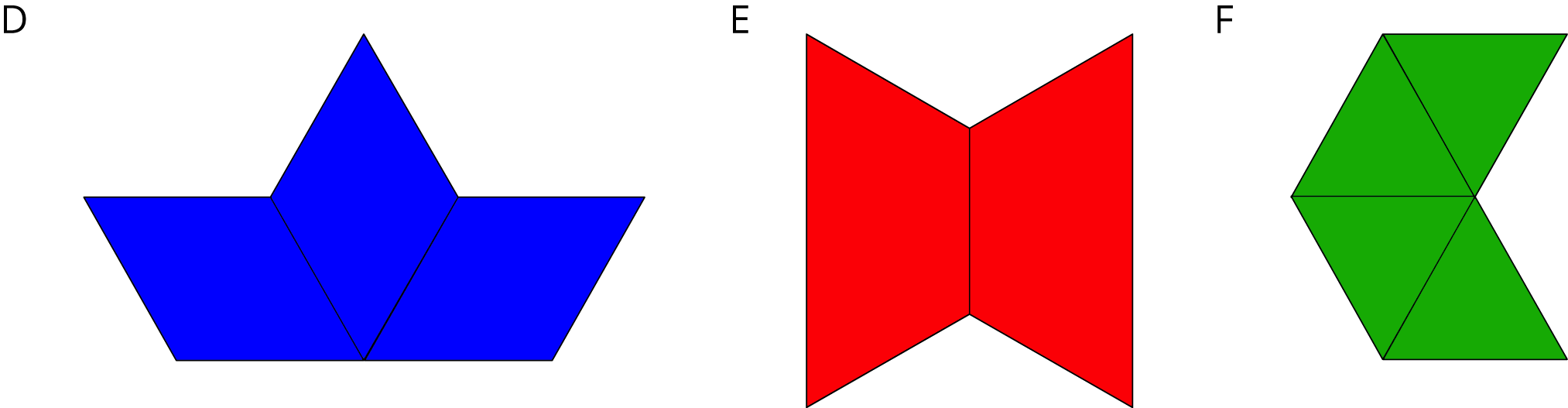
6.1: Scaling a Pattern Block

Use the applets to explore the pattern blocks. Work with your group to build the scaled copies described in each question.

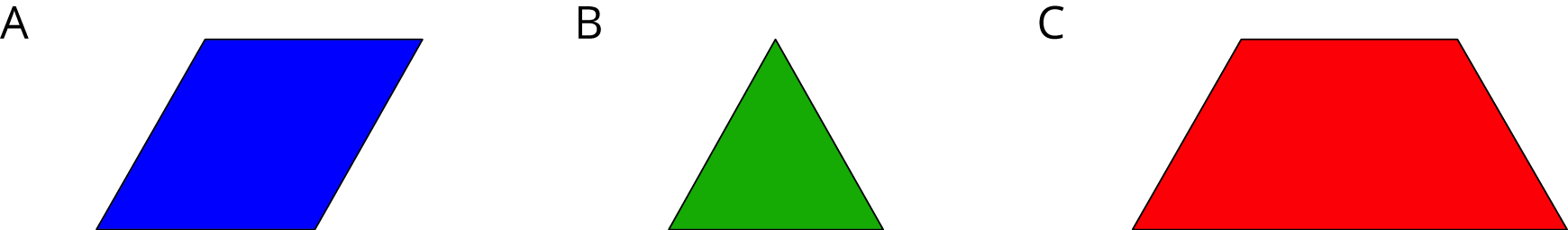
  1. How many blue rhombus blocks does it take to build a scaled copy of Figure A:

    1. Where each side is twice as long?

    2. Where each side is 3 times as long?

    3. Where each side is 4 times as long?

    GeoGebra Applet bGdTdPJR

  2. How many green triangle blocks does it take to build a scaled copy of Figure B:

    1. Where each side is twice as long?

    2. Where each side is 3 times as long?

    3. Using a scale factor of 4?

    GeoGebra Applet KHN97EWB

  3. How many red trapezoid blocks does it take to build a scaled copy of Figure C:

    1. Using a scale factor of 2?

    2. Using a scale factor of 3?

    3. Using a scale factor of 4?

    GeoGebra Applet JAThv7n5

  4. Make a prediction: How many blocks would it take to build scaled copies of these shapes using a scale factor of 5? Using a scale factor of 6? Be prepared to explain your reasoning.

6.2: Scaling More Pattern Blocks

Your teacher will assign your group one of these figures, each made with original-size blocks.

  1. In the applet, move the slider to see a scaled copy of your assigned shape, using a scale factor of 2. Use the original-size blocks to build a figure to match it. How many blocks did it take?

  2. Explain why doubling the blocks was not enough to match the scaled copy, even though the scale factor was 2.

  3. Move the slider to see a scaled copy of your assigned shape using a scale factor of 3. Start building a figure with the original-size blocks to match it. Stop when you can tell for sure how many blocks it would take. Record your answer.

  4. Predict: How many blocks would it take to build scaled copies using scale factors 4, 5, and 6? Explain or show your reasoning.

  5. How is the pattern in this activity the same as the pattern you saw in the previous activity? How is it different?

  6. Discuss your answers with another group that worked on the same shape until you reach an agreement. Be prepared to share your reasoning with the class.

GeoGebra Applet H4FrjARN

GeoGebra Applet fPeQGtej

GeoGebra Applet UseSMZSp

6.3: Area of Scaled Parallelograms and Triangles

  1. Your teacher will give you a figure with measurements in centimeters. What is the area of your figure? How do you know?
  2. Work with your partner to draw scaled copies of your figure, using each scale factor in the table. Complete the table with the measurements of your scaled copies.
    row 1 scale factor base (cm) height (cm) area (cm2)
    row 2 1      
    row 3 2      
    row 4 3      
    row 5 $\frac{1}{2}$      
    row 6 $\frac{1}{3}$      
  3. Compare your results with a group that worked with a different figure. What is the same about your answers? What is different?
  4. If you drew scaled copies of your figure with the following scale factors, what would their areas be? Discuss your thinking. If you disagree, work to reach an agreement. Be prepared to explain your reasoning.
    row 1 scale factor area (cm2)
    row 2 5  
    row 3 $\frac{3}{5}$  

Summary

Scaling affects lengths and areas differently. When we make a scaled copy, all original lengths are multiplied by the scale factor. If we make a copy of a rectangle with side lengths 2 units and 4 units using a scale factor of 3, the side lengths of the copy will be 6 units and 12 units, because $2\boldcdot 3 = 6$ and $4\boldcdot 3 = 12$.

Two rectangles: The first rectangle has a horizontal length labeled 4 and vertical width labeled 2. The second rectangle has a horizontal length labeled 12 and vertical width labeled 6.

The area of the copy, however, changes by a factor of (scale factor)2. If each side length of the copy is 3 times longer than the original side length, then the area of the copy will be 9 times the area of the original, because $3\boldcdot 3$, or $3^2$, equals 9.

Two rectangles. The first rectangle has the vertical side labeled 2 and the horizontal side labeled 4. The second rectangle has the vertical side labeled 6 and the horizontal side labeled 12. Two horizontal dashed lines and 2 vertical dashed lines are drawn in the second rectangle dividing it into 9 identical smaller rectangles.

In this example, the area of the original rectangle is 8 units2 and the area of the scaled copy is 72 units2, because $9\boldcdot 8 = 72$. We can see that the large rectangle is covered by 9 copies of the small rectangle, without gaps or overlaps. We can also verify this by multiplying the side lengths of the large rectangle: $6\boldcdot 12=72$.

Lengths are one-dimensional, so in a scaled copy, they change by the scale factor. Area is two-dimensional, so it changes by the square of the scale factor. We can see this is true for a rectangle with length $l$ and width $w$. If we scale the rectangle by a scale factor of $s$, we get a rectangle with length $s\boldcdot l$ and width $s\boldcdot w$. The area of the scaled rectangle is $A = (s\boldcdot l) \boldcdot (s\boldcdot w)$, so $A= (s^2) \boldcdot (l \boldcdot w)$. The fact that the area is multiplied by the square of the scale factor is true for scaled copies of other two-dimensional figures too, not just for rectangles.

Practice Problems ▶