Let’s use reasoning about rigid transformations to find measurements without measuring.
Here is a triangle.
What can you say about the measures of angles $B$ and $C$?
Here is triangle $ABC$.
Draw midpoint $M$ of side $AC$.
Rotate triangle $ABC$ 180 degrees using center $M$ to form triangle $CDA$. Draw and label this triangle.
In the activity, we made a parallelogram by taking a triangle and its image under a 180-degree rotation around the midpoint of a side. This picture helps you justify a well-known formula for the area of a triangle. What is the formula and how does the figure help justify it?
The picture shows 3 triangles. Triangle 2 and Triangle 3 are images of Triangle 1 under rigid transformations.
Describe a rigid transformation that takes Triangle 1 to Triangle 2. What points in Triangle 2 correspond to points $A$, $B$, and $C$ in the original triangle?
Describe a rigid transformation that takes Triangle 1 to Triangle 3. What points in Triangle 3 correspond to points $A$, $B$, and $C$ in the original triangle?
Find two pairs of line segments in the diagram that are the same length, and explain how you know they are the same length.
Find two pairs of angles in the diagram that have the same measure, and explain how you know they have the same measure.
Here is isosceles triangle $ONE$. Its sides $ON$ and $OE$ have equal lengths. Angle $O$ is 30 degrees. The length of $ON$ is 5 units.
Reflect triangle $ONE$ across segment $ON$. Label the new vertex $M$.
What is the measure of angle $MON$?
What is the measure of angle $MOE$?
Reflect triangle $MON$ across segment $OM$. Label the point that corresponds to $N$ as $T$.
How long is $\overline{OT}$? How do you know?
What is the measure of angle $TOE$?
If you continue to reflect each new triangle this way to make a pattern, what will the pattern look like?
Earlier, we learned that if we apply a sequence of rigid transformations to a figure, then corresponding sides have equal length and corresponding angles have equal measure. These facts let us figure out things without having to measure them!
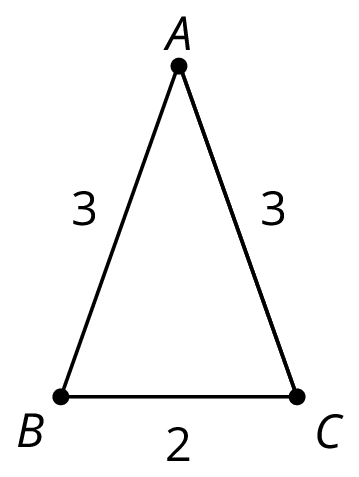
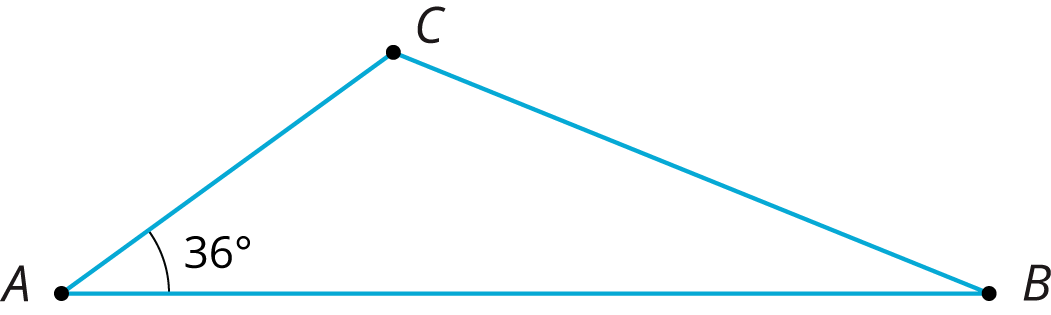
For example, here is triangle $ABC$.
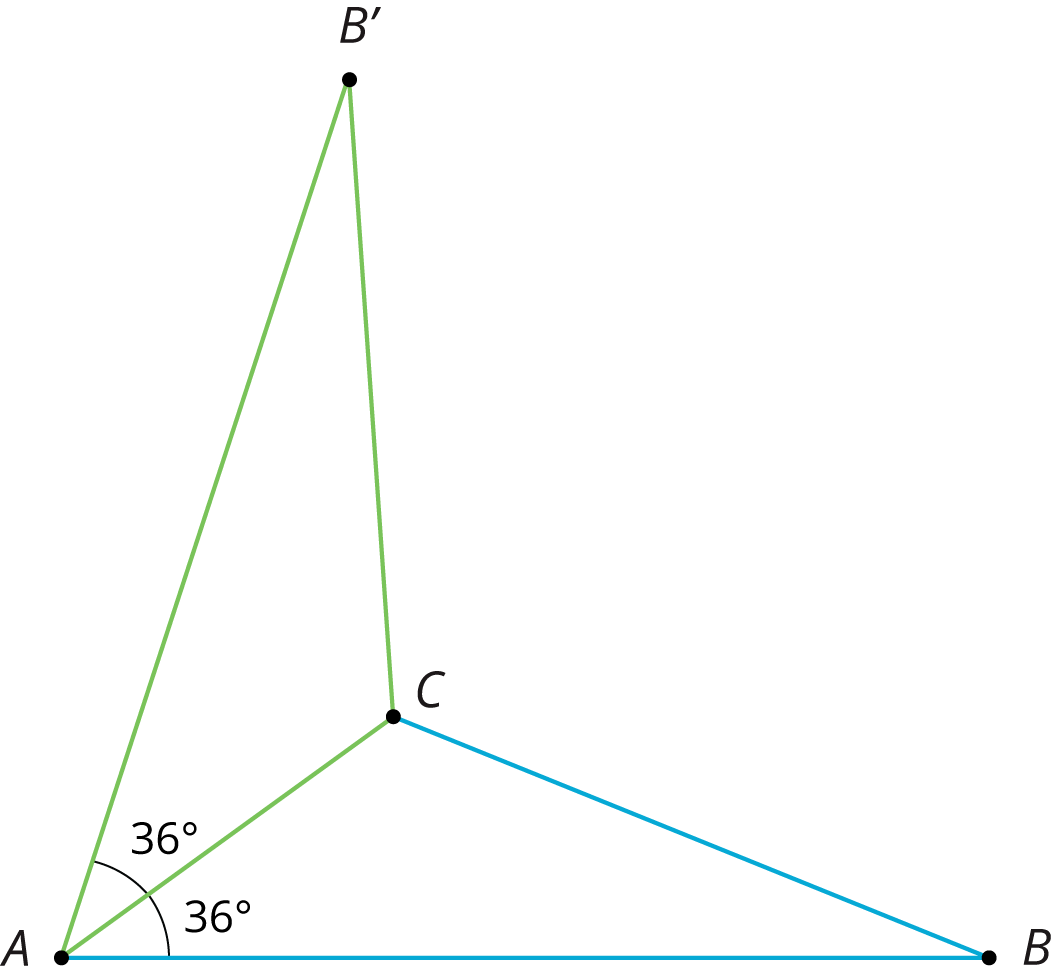
We can reflect triangle $ABC$ across side $AC$ to form a new triangle:
Because points $A$ and $C$ are on the line of reflection, they do not move. So the image of triangle $ABC$ is $AB'C$. We also know that:
When we construct figures using copies of a figure made with rigid transformations, we know that the measures of the images of segments and angles will be equal to the measures of the original segments and angles.
