Lesson 17: Scaling One Dimension

Let’s see how changing one dimension changes the volume of a shape.

17.1: Driving the Distance

Here is a graph of the amount of gas burned during a trip by a tractor-trailer truck as it drives at a constant speed down a highway:

  1. At the end of the trip, how far did the truck drive, and how much gas did it use?
  2. If a truck traveled half this distance at the same rate, how much gas would it use?
  3. If a truck traveled double this distance at the same rate, how much gas would it use?
  4. Complete the sentence: ___________ is a function of _____________.
     

A line is graphed in the coordinate plane with the origin labeled "O." The horizontal axis is labeled "distance traveled in miles" and the numbers 0 through 240, in increments of 40, are indicated. The vertical axis is labeled "gas burned in gallons" and the numbers 0 through 100, in increments of 10, are indicated. The line begins at the origin. It moves slants upward and to the right passing through the coordinates 80 comma 10, 160 comma 20, and ends at the point 240 comma 30.

17.2: Double the Edge

There are many right rectangular prisms with one side of length 5 units and another side of length 3 units. Let $s$ represent the length of the third side and $V$ represent the volume of these prisms.

  1. Write an equation that represents the relationship between $V$ and $s$.

  2. Graph this equation.

     
  3. What happens to the volume if you double the side length $s$? Where do you see this in the graph? Where do you see it algebraically?

17.3: Halve the Height

There are many cylinders with radius 5 units. Let $h$ represent the height and $V$ represent the volume of these cylinders.

  1. Write an equation that represents the relationship between $V$ and $h$. Use 3.14 as an approximation of $\pi$.

  2. Graph this equation.

     
  3. What happens to the volume if you halve the height, $h$? Where can you see this in the graph? How can you see it algebraically?

17.4: Figuring Out Cone Dimensions

Here is a graph of the relationship between the height and the volume of some cones that all have the same radius:

A line is graphed in the coordinate plane with the origin labeled “O”. The horizontal axis is labeled “height of cone” and the numbers 0 through 12 are indicated. The vertical axis is labeled “volume of cone” and the numbers 0 through 4000, in increments of 1000, are indicated. The line begins at the origin. It moves steadily upward and to the right passing through the point that is labeled 10 comma 2355.
  1. What do the coordinates of the labeled point represent?
  2. What is the volume of the cone with height 5? With height 30?
  3. Use the labeled point to find the radius of these cones.  Use 3.14 as an approximation for $\pi$.
  4. Write an equation that relates the volume $V$ and height $h$.

Summary

Imagine a cylinder with a radius of 5 cm that is being filled with water. As the height of the water increases, the volume of water increases.

We say that the volume of the water in the cylinder, $V$, depends on the height of the water $h$. We can represent this relationship with an equation: $V= \pi \boldcdot 5^2h$ or just

$$V = 25\pi h$$

This equation represents a proportional relationship between the height and the volume. We can use this equation to understand how the volume changes when the height is tripled.

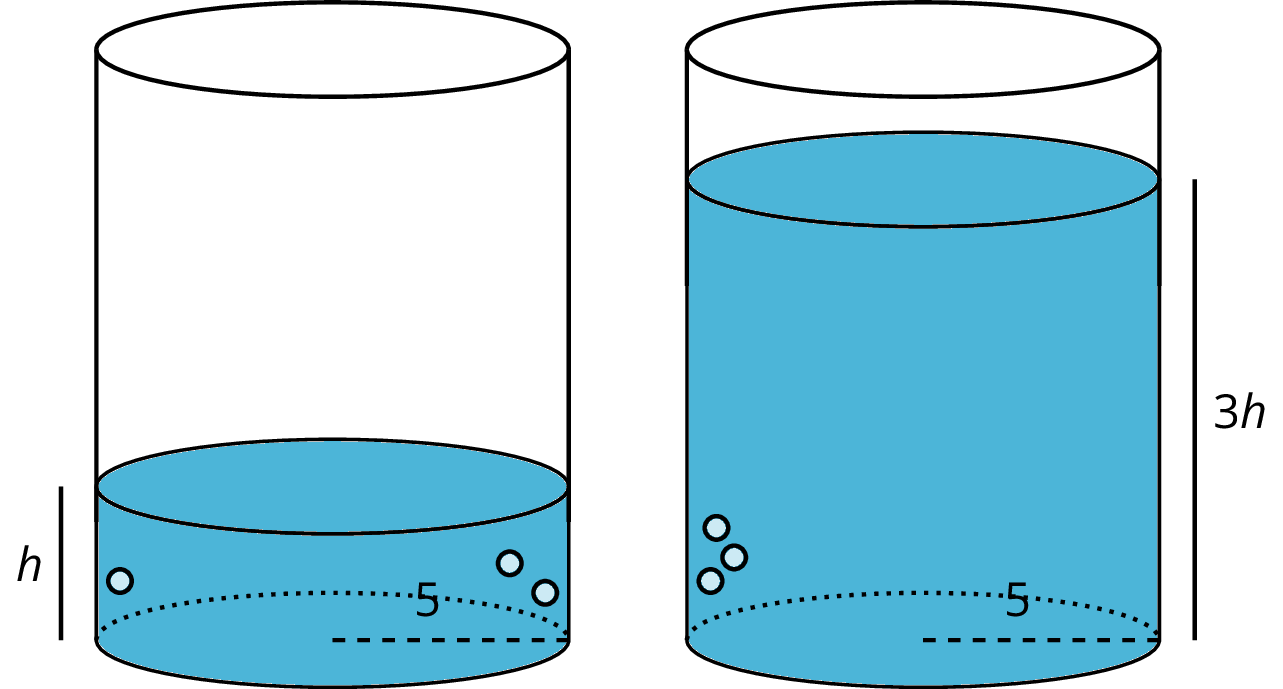
Two identical right circular cylinders with different amounts of shading. The cylinder on the left has a radius labeled 5. The height of the shading in the cylinder on the left is labeled h. The cylinder on the right has a radius labeled 5. The height of the shading in the cylinder on the right is labeled "three h."

The new volume would be $V = 25 \pi (3h) = 75 \pi h$, which is precisely 3 times as much as the old volume of $25\pi h$. In general, when one quantity in a proportional relationship changes by a given factor, the other quantity changes by the same factor.

Remember that proportional relationships are examples of linear relationships, which can also be thought of as functions. So in this example $V$, the volume of water in the cylinder, is a function of the height $h$ of the water.

Practice Problems ▶