Lesson 5: Introduction to Linear Relationships

Let’s explore some relationships between two variables.

5.1: Number Talk: Fraction Division

Find the value of $2\frac58 \div \frac12$.

5.2: Stacking Cups

We have two stacks of styrofoam cups. One stack has 6 cups, and its height is 15 cm. The other one has 12 cups, and its height is 23 cm. How many cups are needed for a stack with a height of 50 cm?

5.3: Connecting Slope to Rate of Change

  1. If you didn’t create your own graph of the situation before, do so now.
  2. What are some ways you can tell that the number of cups is not proportional to the height of the stack?
  3. What is the slope of the line in your graph? What does the slope mean in this situation?
  4. At what point does your line intersect the vertical axis? What do the coordinates of this point tell you about the cups?
  5. How much height does each cup after the first add to the stack?
     

Summary

Andre starts babysitting and charges \$10 for traveling to and from the job, and \$15 per hour. For every additional hour he works he charges another \$15. If we graph Andre's earnings based on how long he works, we have a line that starts at \$10 on the vertical axis and then increases by \$15 each hour. A linear relationship is any relationship between two quantities where one quantity has a constant rate of change with respect to the other. 

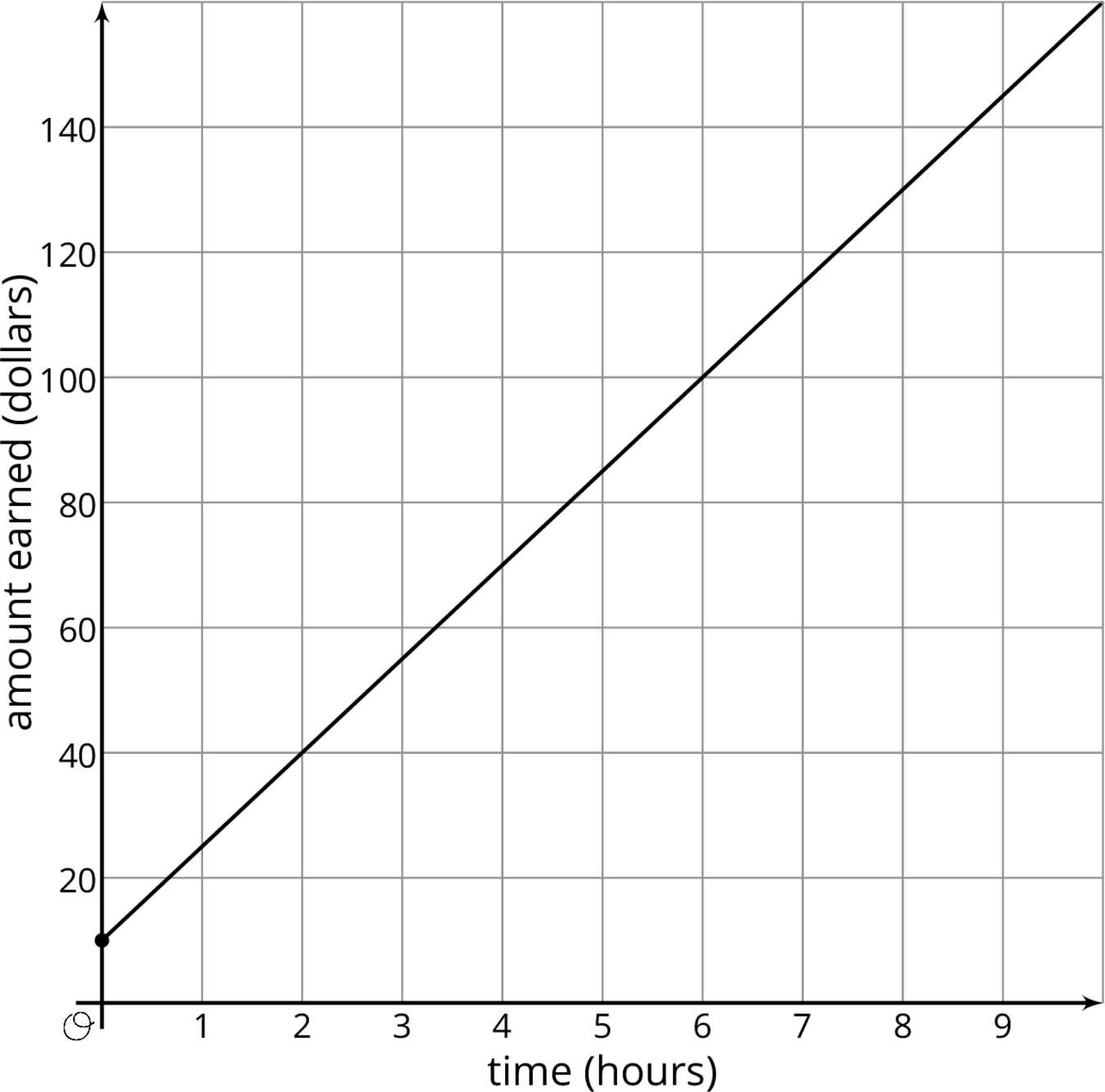
We can figure out the rate of change using the graph. Because the rate of change is constant, we can take any two points on the graph and divide the amount of vertical change by the amount of horizontal change. For example, take the points $(2, 40)$ and $(6, 100)$. They mean that Andre earns \$40 for working 2 hours and \$100 for working 6 hours. The rate of change is $\frac{100-40}{6-2} = 15$ dollars per hour. Andre's earnings go up \$15 for each hour of babysitting. Notice that this is the same way we calculate the slope of the line. That's why the graph is a line, and why we call this a linear relationship. The rate of change of a linear relationship is the same as the slope of its graph.

With proportional relationships we are used to graphs that contain the point $(0,0)$. But proportional relationships are just one type of linear relationship. In the following lessons, we will continue to explore the other type of linear relationship where the quantities are not both 0 at the same time.

Practice Problems ▶

Glossary

slope

slope

The slope of a line is the quotient of the vertical distance and the horizontal distance between any two points on the line.

rate of change

rate of change

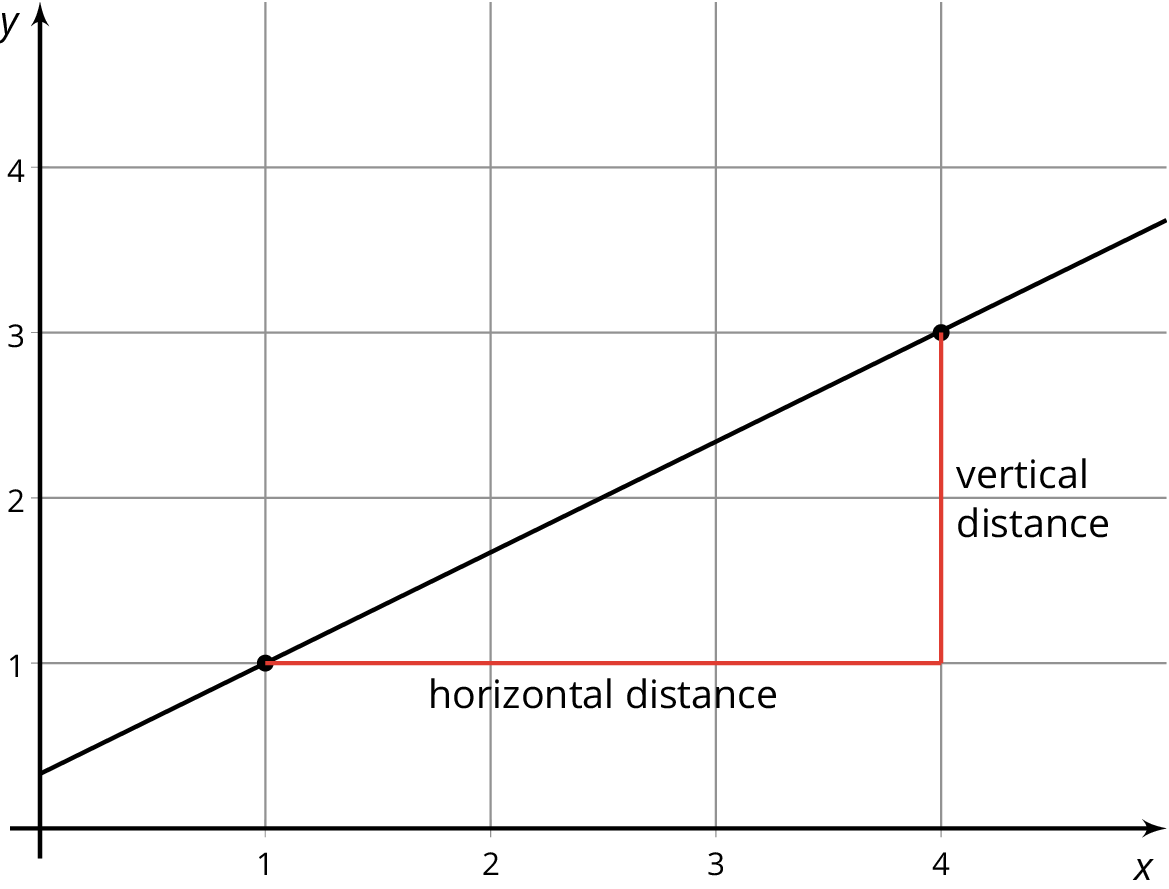
In a linear relationship between two quantities $x$ and $y$, with equation $y = mx + b$,  the constant $m$ is the rate of change. It tells you how much $y$ changes when $x$ changes by 1. It is also the slope of the graph of the relationship.

linear relationship

linear relationship

A linear relationship is a relationship between two quantities where one quantity has a constant rate of change with respect to the other. The relationship is called linear because its graph is a line. A linear relationship can be represented by an equation of the form $y = mx + b$, where $m$ and $b$ are constants.