Lesson 10: Calculating Slope

Let’s calculate slope from two points.

10.1: Integer Operations Review

Find values for $a$ and $b$ that make each side have the same value.

  1. $\frac{a}{b}=\text-2$
  2. $\frac{a}{b}=2$
  3. $a-b=\text-2$

10.2: Toward a More General Slope Formula

  1. Plot the points $(1,11)$ and $(8, 2)$, and use a ruler to draw the line that passes through them.

  1. Without calculating, do you expect the slope of the line through $(1,11)$ and $(8, 2)$ to be positive or negative? How can you tell?

  2. Calculate the slope of this line.

10.3: Making Designs

Your teacher will give you either a design or a blank graph. Do not show your card to your partner.

If your teacher gives you the design:

  1. Look at the design silently and think about how you could communicate what your partner should draw. Think about ways that you can describe what a line looks like, such as its slope or points that it goes through.
  2. Describe each line, one at a time, and give your partner time to draw them.
  3. Once your partner thinks they have drawn all the lines you described, only then should you show them the design.

If your teacher gives you the blank graph:

  1. Listen carefully as your partner describes each line, and draw each line based on their description.
  2. You are not allowed to ask for more information about a line than what your partner tells you.
  3. Do not show your drawing to your partner until you have finished drawing all the lines they describe.

When finished, place the drawing next to the card with the design so that you and your partner can both see them. How is the drawing the same as the design? How is it different? Discuss any miscommunication that might have caused the drawing to look different from the design.

Pause here so your teacher can review your work. When your teacher gives you a new set of cards, switch roles for the second problem.

Summary

We learned earlier that one way to find the slope of a line is by drawing a slope triangle. For example, using the slope triangle shown here, the slope of the line is $\text{-}\frac24$, or $\text{-}\frac12$ (we know the slope is negative because the line is decreasing from left to right).

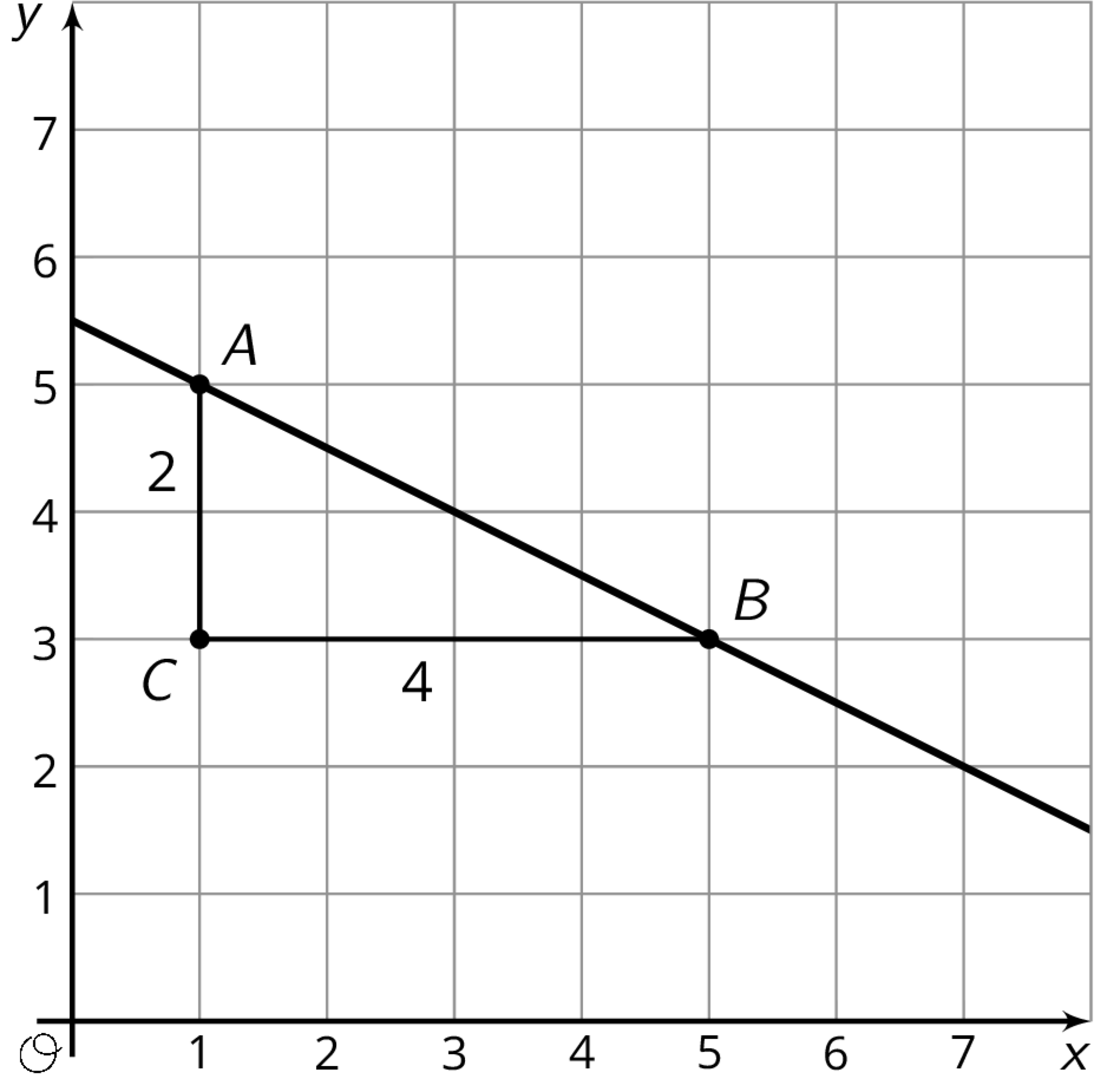
But slope triangles are only one way to calculate the slope of a line. Let’s compute the slope of this line a different way using just the points $A=(1,5)$ and $B=(5,3)$. Since we know the slope is the vertical change divided by the horizontal change, we can calculate the change in the $y$-values and then the change in the $x$-values. Between points $A$ and $B$, the $y$-value change is $3-5=\text{-}2$ and the $x$-value change is $5-1=4$. This means the slope is $\text{-}\frac24$, or $\text{-}\frac12$, which is the same as what we found using the slope triangle.

Notice that in each of the calculations, We subtracted the value from point $A$ from the value from point $B$. If we had done it the other way around, then the $y$-value change would have been $5-3=2$ and the $x$-value change would have been $1-5=\text-4$, which still gives us a slope of $\text- \frac12$. But what if we were to mix up the orders? If that had happened, we would think the slope of the line is positive $\frac12$ since we would either have calculated $\frac{\text-2}{\text-4}$ or $\frac{2}{4}$. Since we already have a graph of the line and can see it has a negative slope, this is clearly incorrect. It we don’t have a graph to check our calculation, we could think about how the point on the left, $(1,5)$, is higher than the point on the right, $(5,3)$, meaning the slope of the line must be negative.

Practice Problems ▶