12.1: Translated Images
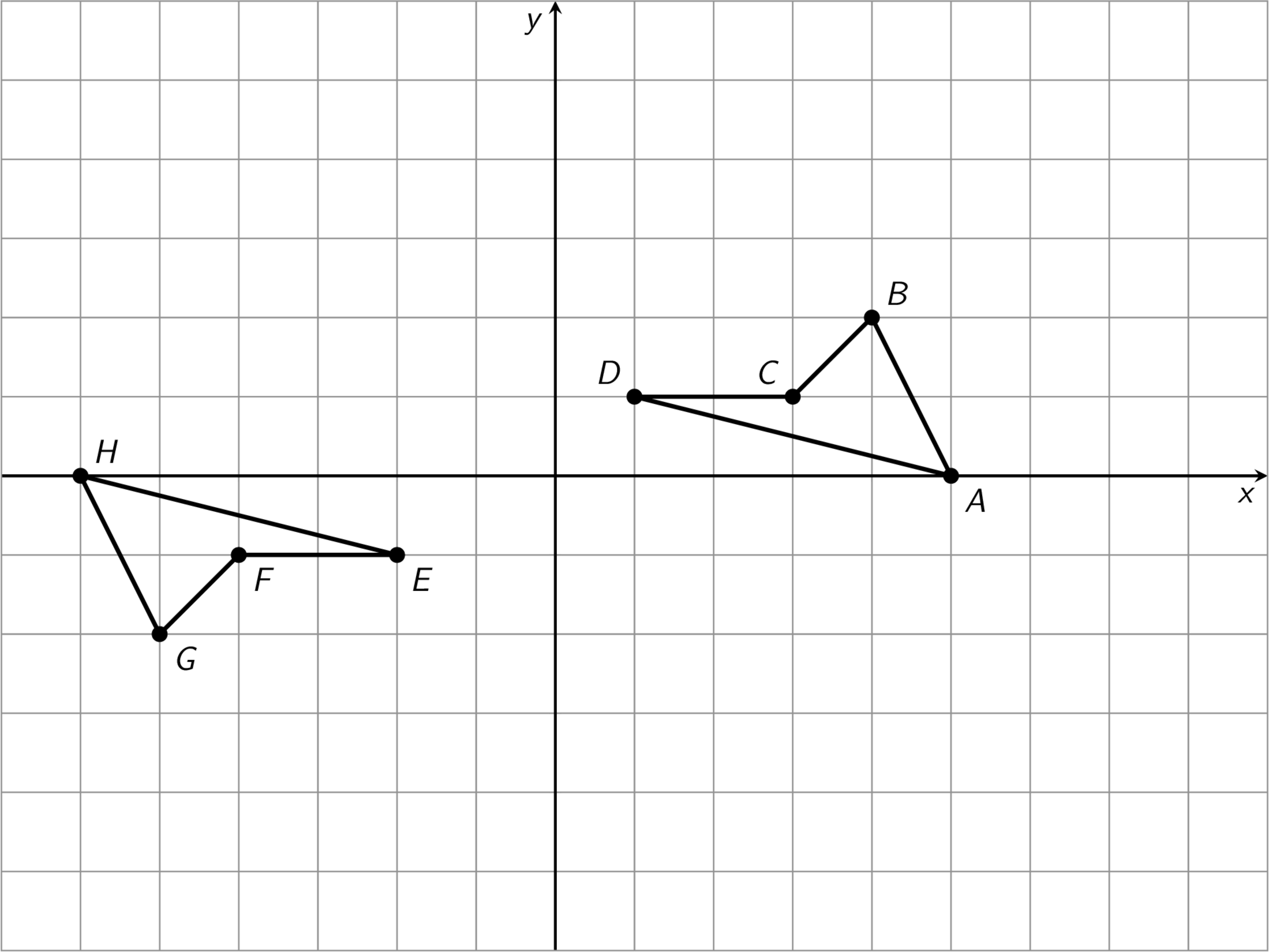
All of these triangles are congruent. Sometimes we can take one figure to another with a translation. Shade the triangles that are images of triangle $ABC$ under a translation.

Let’s decide if two figures are congruent.
All of these triangles are congruent. Sometimes we can take one figure to another with a translation. Shade the triangles that are images of triangle $ABC$ under a translation.

For each of the following pairs of shapes, decide whether or not they are congruent. Explain your reasoning.
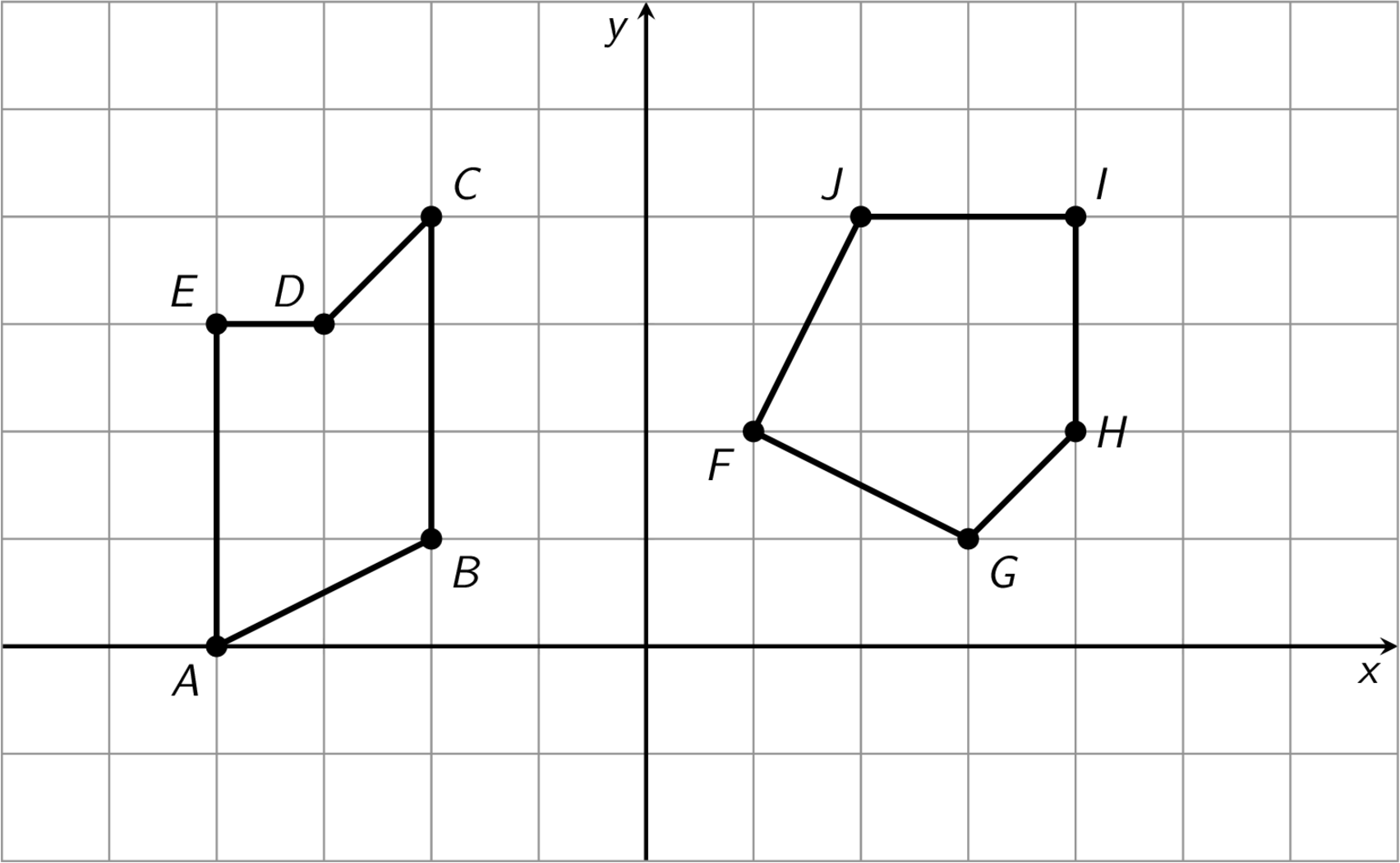
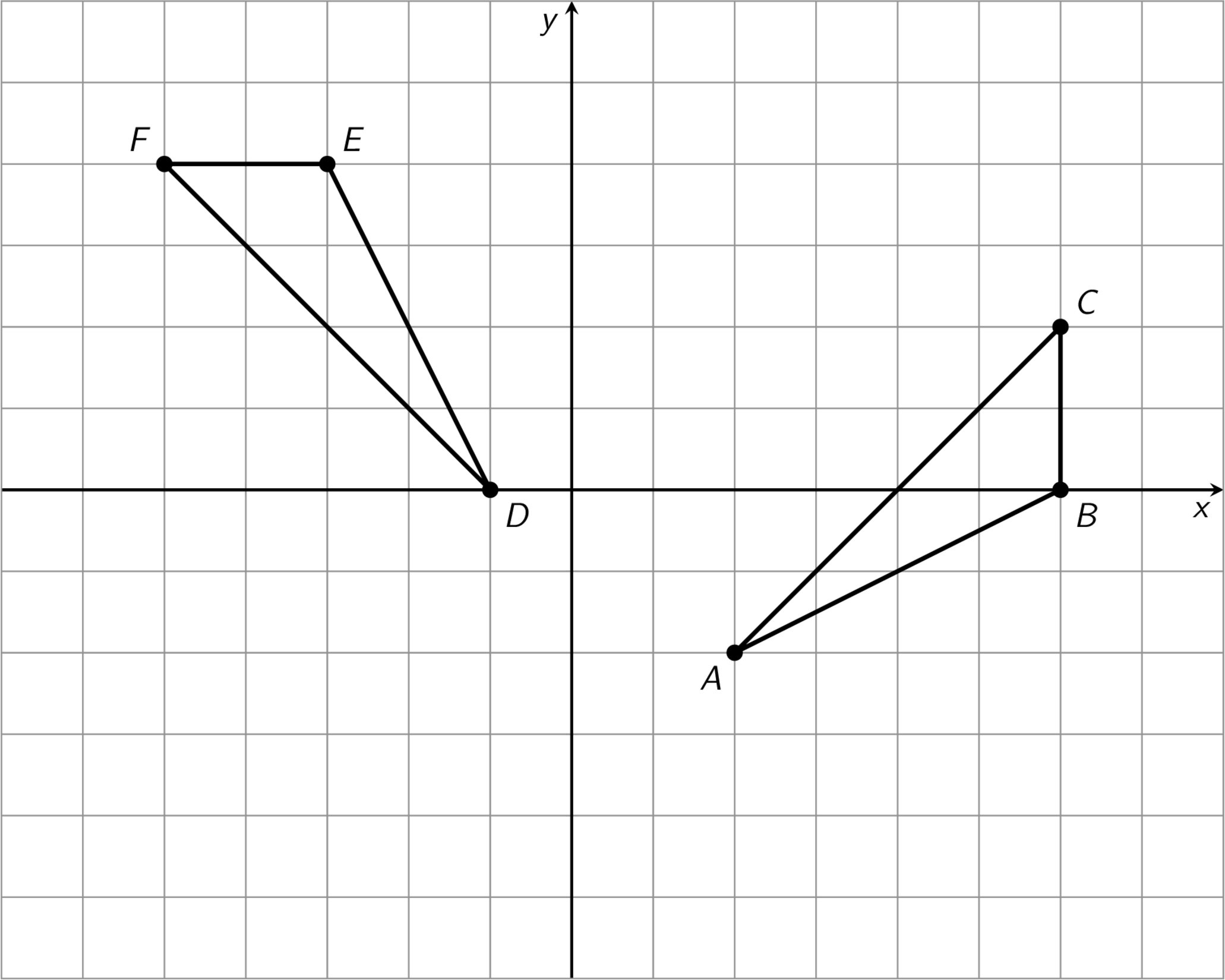
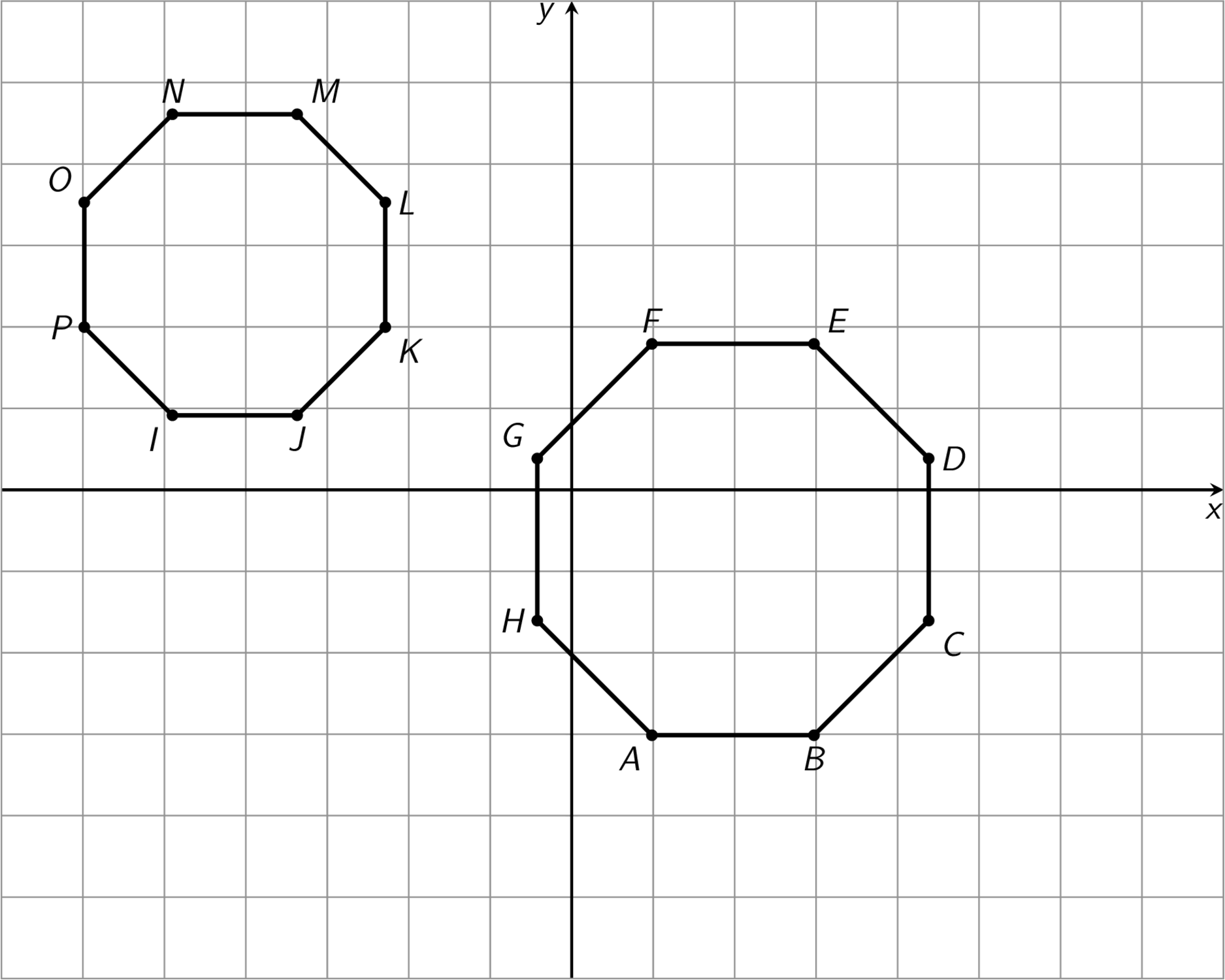
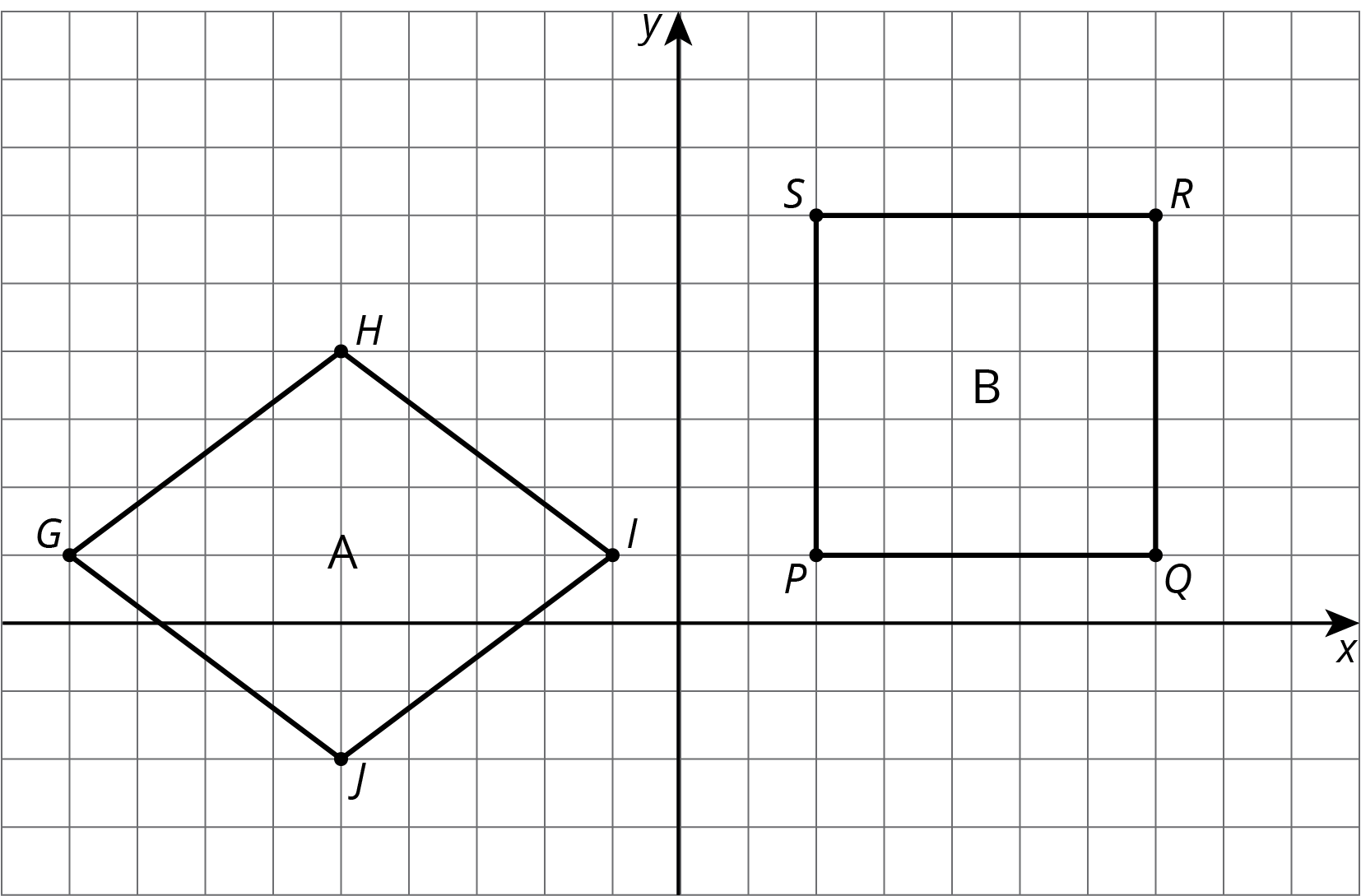
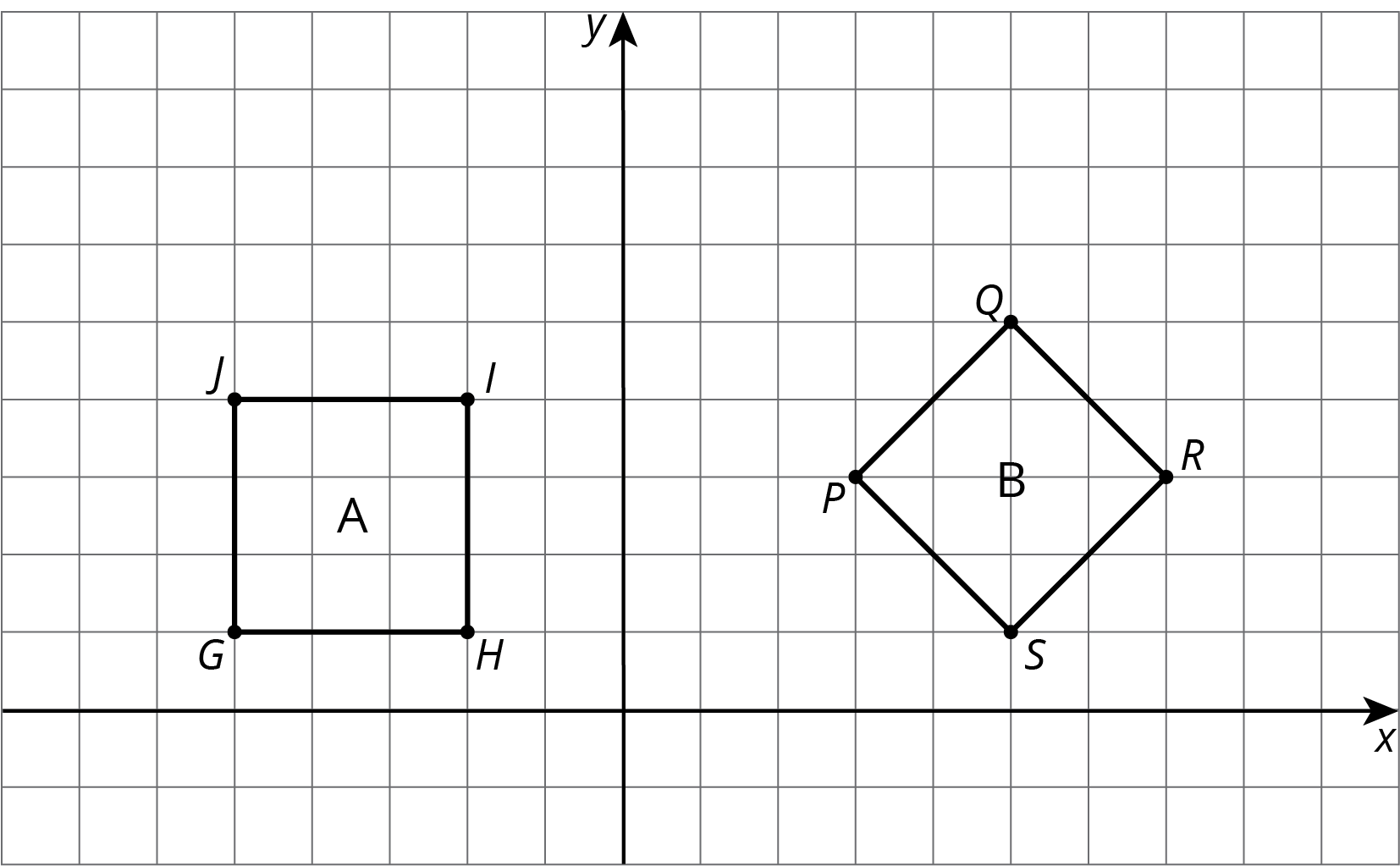
For each pair of shapes, decide whether or not Shape A is congruent to Shape B. Explain how you know.
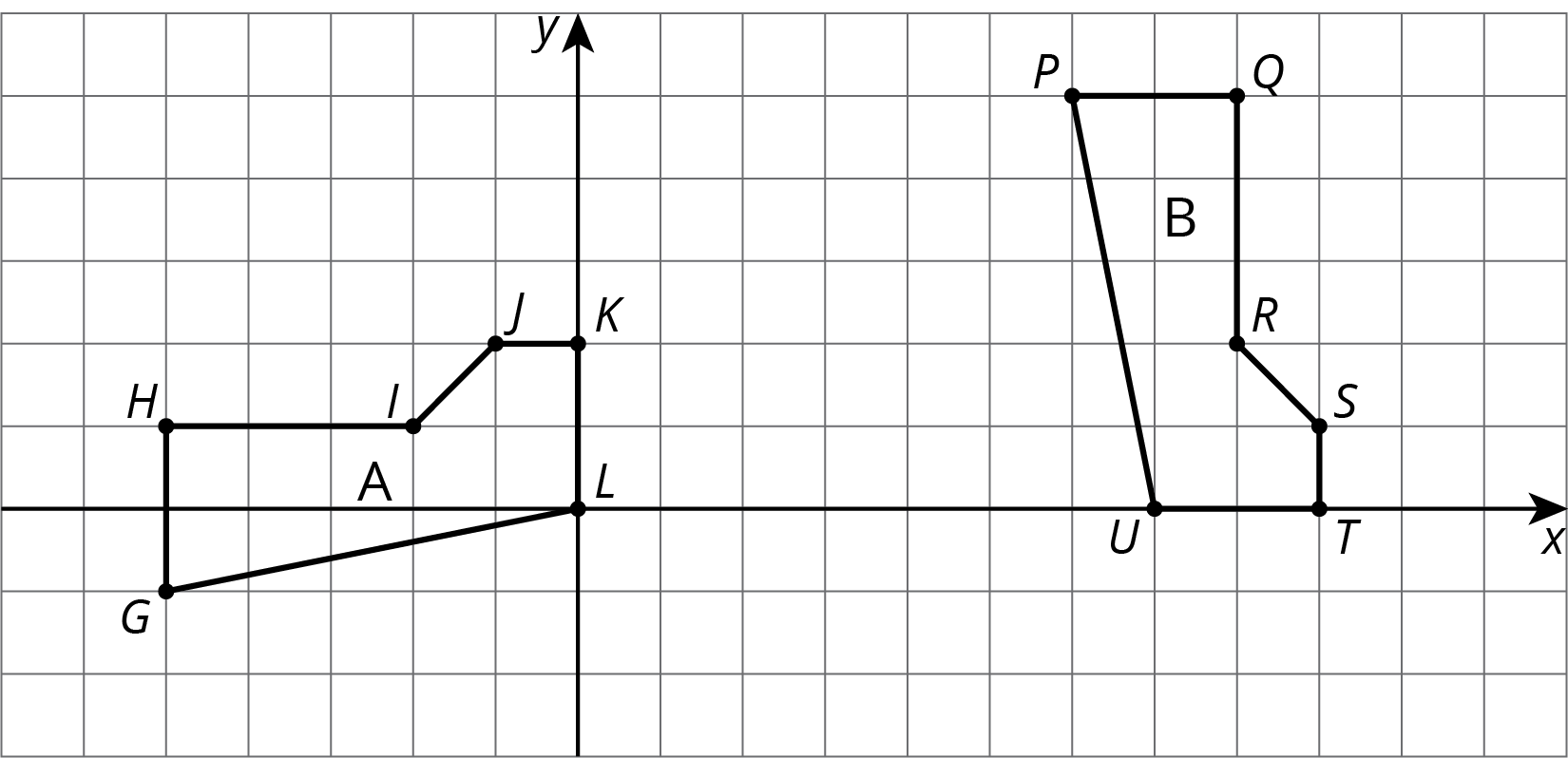
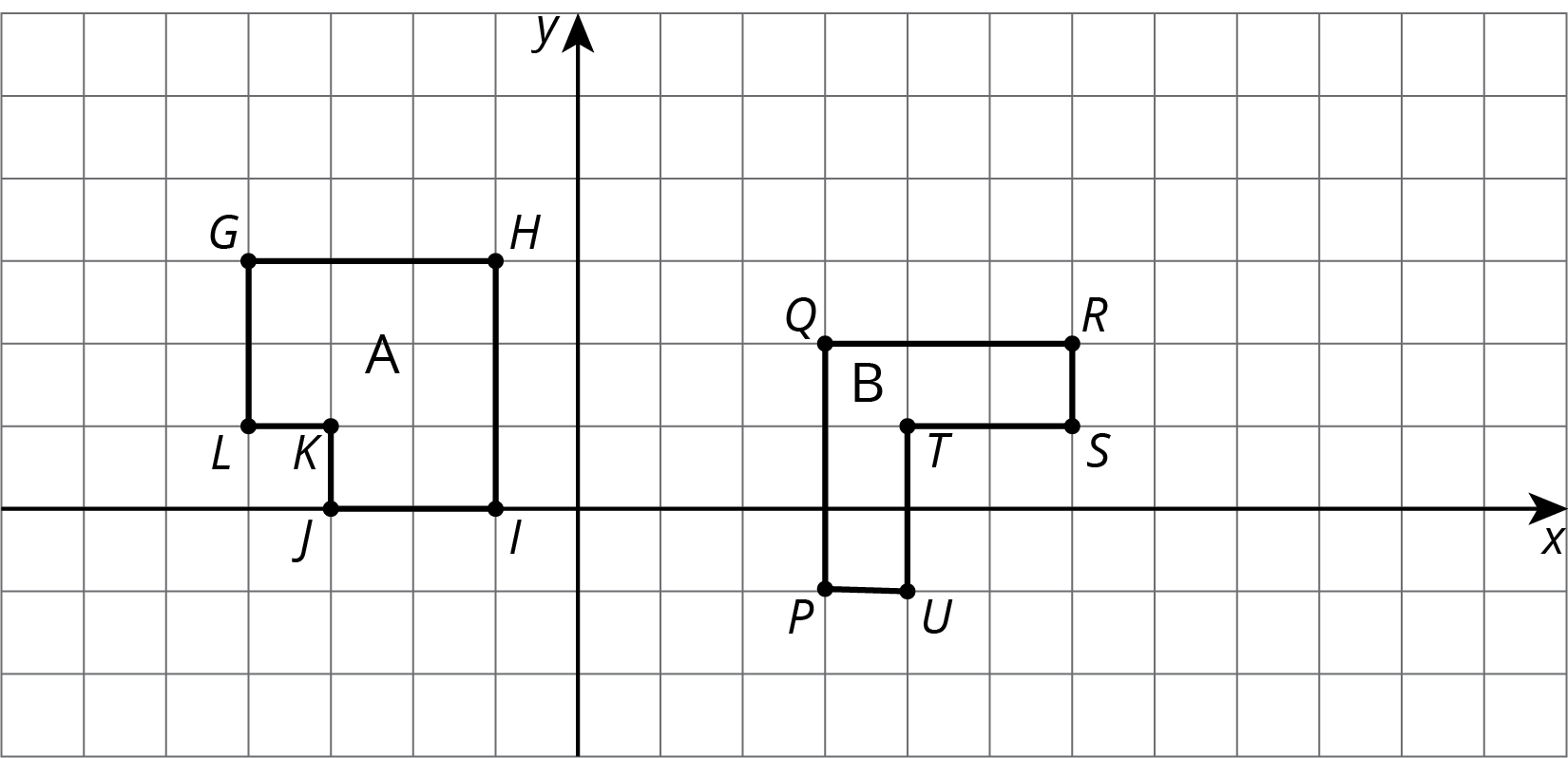
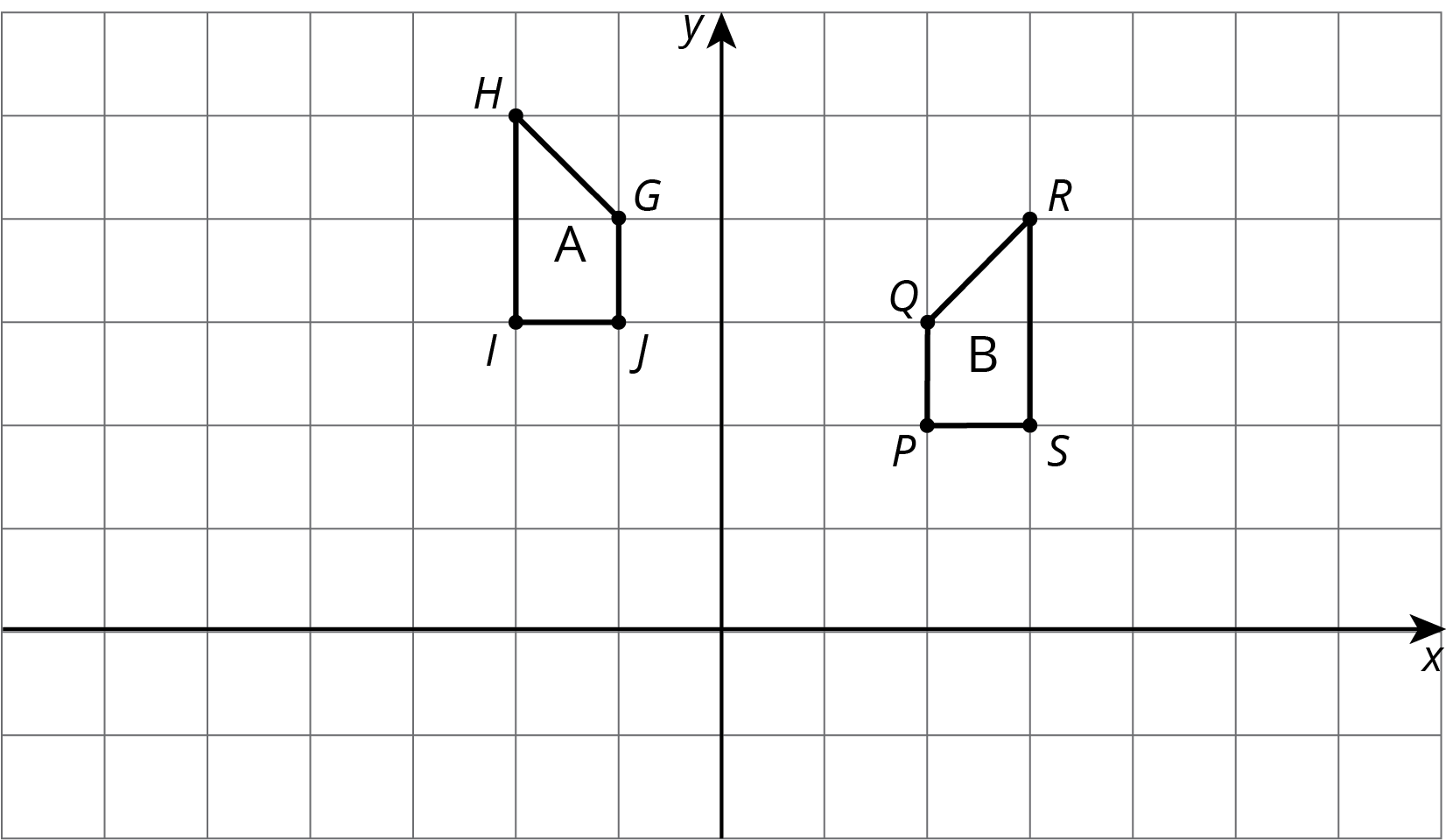
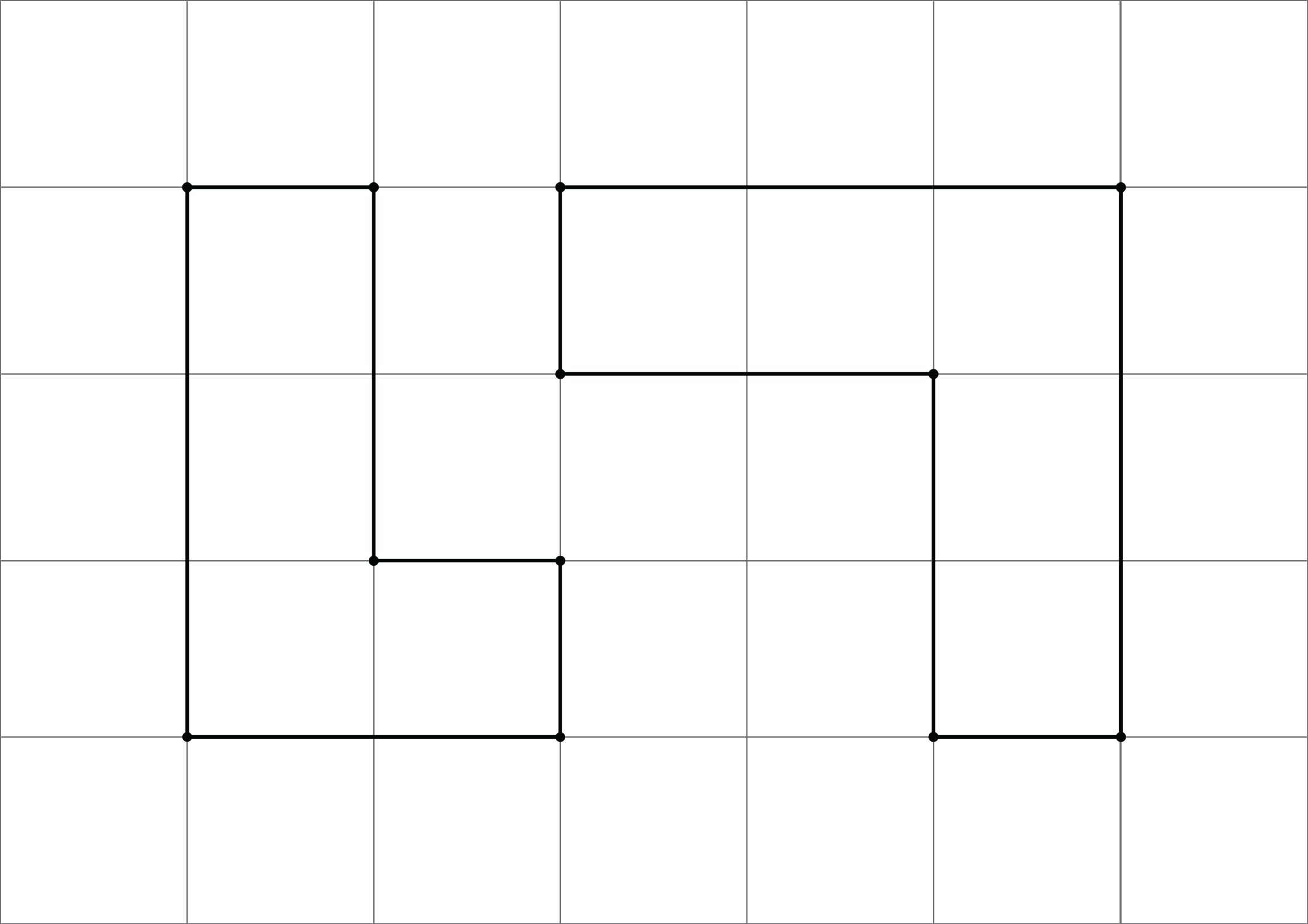
A polygon has 8 sides: five of length 1, two of length 2, and one of length 3. All sides lie on grid lines. (It may be helpful to use graph paper when working on this problem.)
Find a polygon with these properties.
Your teacher will give you a set of four objects.
Compare your quadrilateral with your partner’s. Are they congruent? Explain how you know.
Repeat steps 1 and 2, forming different quadrilaterals. If your first quadrilaterals were not congruent, can you build a pair that is? If your first quadrilaterals were congruent, can you build a pair that is not? Explain.
How do we know if two figures are congruent?
If we copy one figure on tracing paper and move the paper so the copy covers the other figure exactly, then that suggests they are congruent.
How do we know that two figures are not congruent?
If there is no correspondence between the figures where the parts have equal measure, that proves that the two figures are not congruent. In particular,
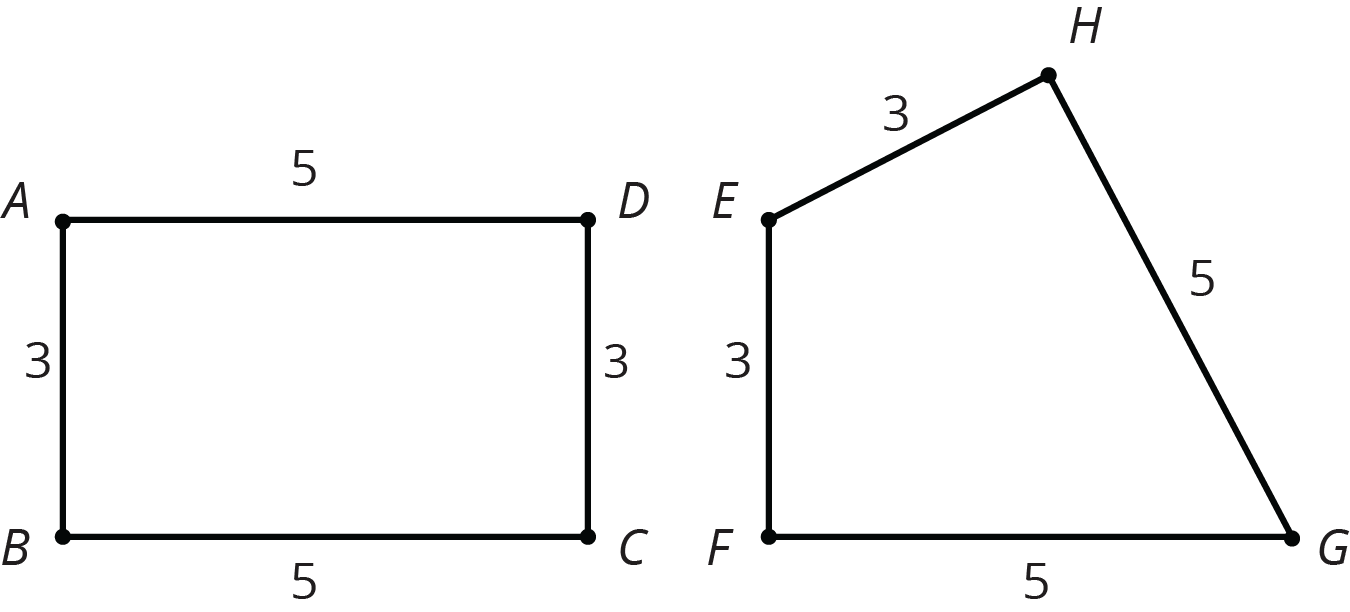
If two polygons have different sets of side lengths, they can’t be congruent. For example, the figure on the left has side lengths 3, 2, 1, 1, 2, 1. The figure on the right has side lengths 3, 3, 1, 2, 2, 1. There is no way to make a correspondence between them where all corresponding sides have the same length.
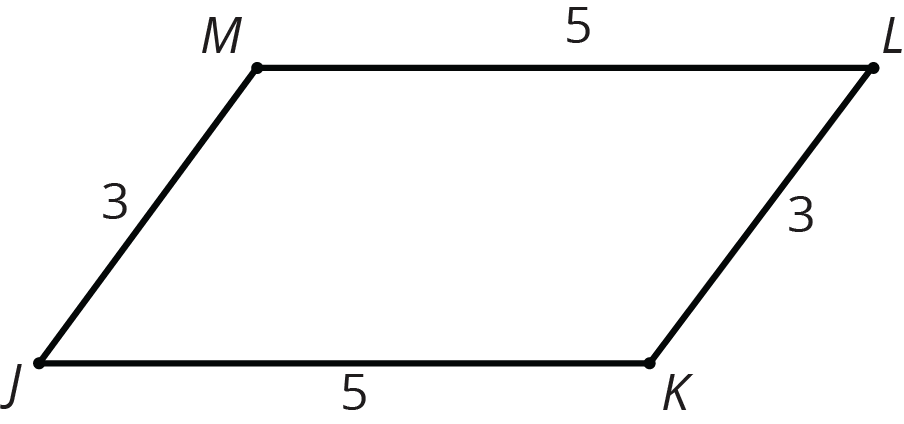
If two polygons have the same side lengths, in the same order, but different corresponding angles, the polygons can’t be congruent. For example, parallelogram $JKLM$ can’t be congruent to rectangle $ABCD$. Even though they have the same side lengths in the same order, the angles are different. All angles in $ABCD$ are right angles. In $JKLM$, angles $J$ and $L$ are less than 90 degrees and angles $K$ and $M$ are more than 90 degrees.