Lesson 21: Cylinders, Cones, and Spheres

Let’s find the volume of shapes.

21.1: Sphere Arguments

Four students each calculated the volume of a sphere with a radius of 9 centimeters and they got four different answers.

  • Han thinks it is 108 cubic centimeters.
  • Jada got $108\pi$ cubic centimeters.
  • Tyler calculated 972 cubic centimeters.
  • Mai says it is $972\pi$ cubic centimeters.

Do you agree with any of them? Explain your reasoning.

21.2: Sphere’s Radius

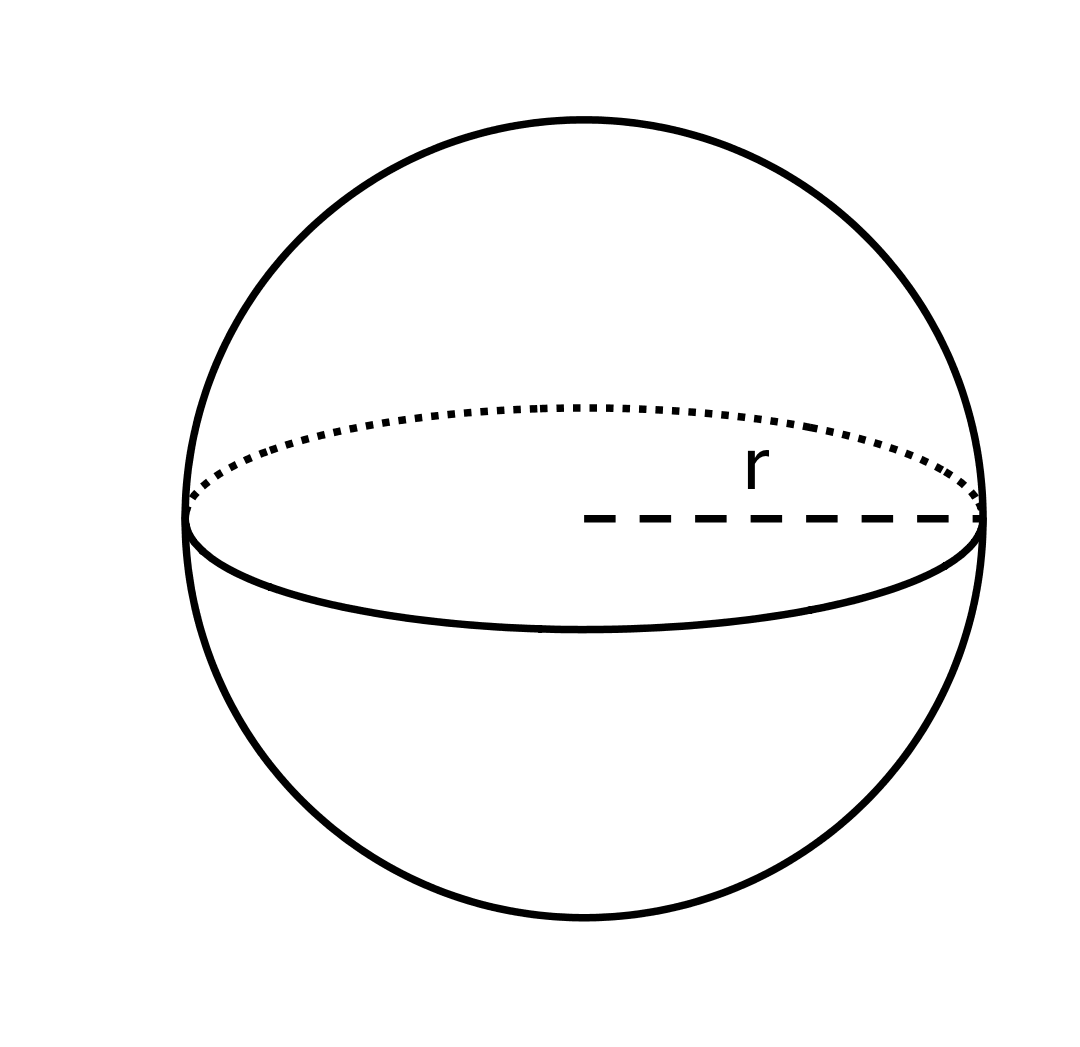
A sphere. A dashed line is drawn from the center of the sphere to the edge of the sphere and is labeled "r."

The volume of this sphere with radius $r$ is $V=288\pi$. This statement is true:

$$288\pi =\frac43 r^3 \pi.$$ What is the value of $r$ for this sphere? Explain how you know.

21.3: Info Gap: Unknown Dimensions

Your teacher will give you either a problem card or a data card. Do not show or read your card to your partner.

If your teacher gives you the problem card:

  1. Silently read your card and think about what information you need to answer the question.
  2. Ask your partner for the specific information that you need.
  3. Explain to your partner how you are using the information to solve the problem.
  4. Solve the problem and explain your reasoning to your partner.

If your teacher gives you the data card:

  1. Silently read the information on your card.
  2. Ask your partner “What specific information do you need?” and wait for your partner to ask for information. Only give information that is on your card. (Do not figure out anything for your partner!)
  3. Before telling your partner the information, ask “Why do you need that information?”
  4. After your partner solves the problem, ask them to explain their reasoning and listen to their explanation.

Pause here so your teacher can review your work. Ask your teacher for a new set of cards and repeat the activity, trading roles with your partner.

21.4: The Right Fit

A right circular cylinder witha diameter of 3 centimeters and a height of 8 centimeters.

A cylinder with diameter 3 centimeters and height 8 centimeters is filled with water. Decide which figures described here, if any, could hold all of the water from the cylinder. Explain your reasoning.

  1. Cone with a height of 8 centimeters and a radius of 3 centimeters.
  2. Cylinder with a diameter of 6 centimeters and height of 2 centimeters.
  3. Rectangular prism with a length of 3 centimeters, width of 4 centimeters, and height of 8 centimeters.
  4. Sphere with a radius of 2 centimeters.

Summary

The formula

$$V=\frac43 \pi r^3$$

gives the volume of a sphere with radius $r$. We can use the formula to find the volume of a sphere with a known radius. For example, if the radius of a sphere is 6 units, then the volume would be

$$\frac{4}{3} \pi (6)^3 = 288\pi$$

or approximately $904$ cubic units. We can also use the formula to find the radius of a sphere if we only know its volume. For example, if we know the volume of a sphere is $36 \pi$ cubic units but we don't know the radius, then this equation is true: 

$$36\pi=\frac43\pi r^3$$

That means that $r^3 = 27$, so the radius $r$ has to be 3 units in order for both sides of the equation to have the same value.

Many common objects, from water bottles to buildings to balloons, are similar in shape to rectangular prisms, cylinders, cones, and spheres—or even combinations of these shapes! Using the volume formulas for these shapes allows us to compare the volume of different types of objects, sometimes with surprising results.

For example, a cube-shaped box with side length 3 centimeters holds less than a sphere with radius 2 centimeters because the volume of the cube is 27 cubic centimeters ($3^3 = 27$), and the volume of the sphere is around 33.51 cubic centimeters ($\frac43\pi \boldcdot  2^3 \approx 33.51$).

Practice Problems ▶