Lesson 13: Solving Systems of Equations

Let’s solve systems of equations.

13.1: True or False: Two Lines

Use the lines to decide whether each statement is true or false. Be prepared to explain your reasoning using the lines.

  1. A solution to $8=\text-x+10$ is 2.
  2. A solution to $2=2x+4$ is 8.
  3. A solution to $\text-x+10=2x+4$ is 8.
  4. A solution to $\text-x+10=2x+4$ is 2.
  5. There are no values of $x$ and $y$ that make $y=\text-x+10$ and $y=2x+4$ true at the same time.

13.2: Matching Graphs to Systems

Here are three systems of equations graphed on a coordinate plane:

  1. Match each figure to one of the systems of equations shown here.

    1. $\begin{cases} y=3x+5\\ y=\text- 2x+20 \end{cases}$

    2. $\begin{cases} y=2x-10\\ y=4x-1 \end{cases}$

    3. $\begin{cases} y=0.5x+12\\ y=2x+27 \end{cases}$

  2. Find the solution to each system and then check that your solution is reasonable on the graph.
    • Notice that the sliders set the values of the coefficient and the constant term in each equation.
    • Change the sliders to the values of the coefficient and the constant term in the next pair of equations.
    • Click on the spot where the lines intersect and a labeled point should appear.

13.3: Different Types of Systems

Your teacher will give you a page with 6 systems of equations.

  1. Graph each system of equations by typing each pair of the equations in the applet, one at a time.

  2. Describe what the graph of a system of equations looks like when it has . . .
    1. 1 solution
    2. 0 solutions
    3. infinitely many solutions
 

Use the applet to confirm your answer to question 2.

Summary

Sometimes it is easier to solve a system of equations without having to graph the equations and look for an intersection point. In general, whenever we are solving a system of equations written as

$$\begin{cases} y = \text{[some stuff]}\\ y = \text{[some other stuff]} \end{cases}$$

we know that we are looking for a pair of values $(x,y)$ that makes both equations true. In particular, we know that the value for $y$ will be the same in both equations. That means that

$$\text{[some stuff]} = \text{[some other stuff]}$$

For example, look at this system of equations:

$$\begin{cases} y = 2x + 6 \\ y  = \text-3x - 4 \end{cases}$$

Since the $y$ value of the solution is the same in both equations, then we know $$2x + 6  = \text-3x -4$$

We can solve this equation for $x$:

\(\begin{align} 2x + 6  &= \text-3x -4 \\ 5x + 6 &= \text-4 && \text{add $3x$ to each side} \\ 5x &= \text-10 && \text{subtract 6 from each side} \\ x &= \text-2 && \text{divide each side by 5} \end{align}\)

But this is only half of what we are looking for: we know the value for $x$, but we need the corresponding value for $y$. Since both equations have the same $y$ value, we can use either equation to find the $y$-value:

$$y = 2(\text-2) + 6$$

Or

$$y = \text-3(\text-2) -4$$

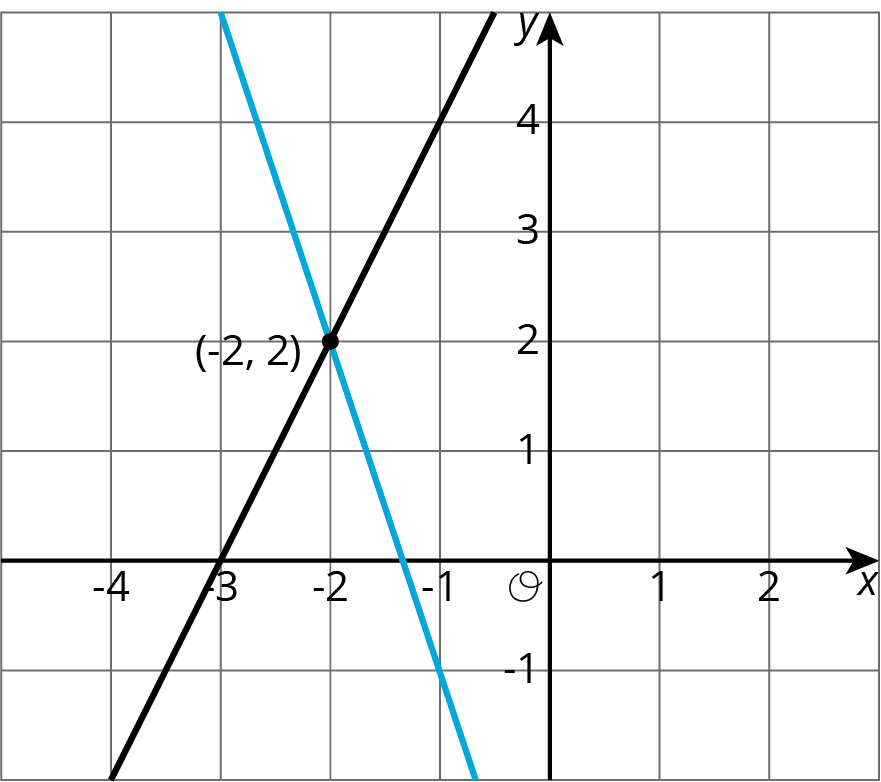
In both cases, we find that $y = 2$. So the solution to the system is $(\text-2,2)$. We can verify this by graphing both equations in the coordinate plane.

In general, a system of linear equations can have:

  • No solutions. In this case, the lines that correspond to each equation never intersect.
  • Exactly one solution. The lines that correspond to each equation intersect in exactly one point.
  • An infinite number of solutions. The graphs of the two equations are the same line!

Practice Problems ▶